CONCEPT
Though people seldom witness it directly, the entire world is in a state of motion, and where solid objects are concerned, this motion is manifested as vibration. When the vibrations produced by one object come into alignment with those of another, this is called resonance. The power of resonance can be as gentle as an adult pushing a child on a swing, or as ferocious as the force that toppled what was once the world’s third-longest suspension bridge. Resonance helps to explain all manner of familiar events, from the feedback produced by an electric guitar to the cooking of food in a microwave oven.
HOW IT WORKS
Vibration of Molecules
The possibility of resonance always exists wherever there is periodic motion, movement that is repeated at regular intervals called periods, and/or harmonic motion, the repeated movement of a particle about a position of equilibrium or balance. Many examples of resonance involve large objects: a glass, a child on a swing, a bridge. But resonance also takes place at a level invisible to the human eye using even the most powerful optical microscope.
All molecules exert a certain electromagnetic attraction toward each other, and generally speaking, the less the attraction between molecules, the greater their motion relative to one another. This, in turn, helps define the object in relation to its particular phase of matter.
A substance in which molecules move at high speeds, and therefore hardly attract one another at all, is called a gas. Liquids are materials in which the rate of motion, and hence of intermolecular attraction, is moderate. In a solid, on the other hand, there is little relative motion, and therefore molecules exert enormous attractive forces. Instead of moving in relation to one another, the molecules that make up a solid tend to vibrate in place.
Due to the high rate of motion in gas molecules, gases possess enormous internal kinetic energy. The internal energy of solids and liquids is much less than in gases, yet, as we shall see, the use of resonance to transfer energy to these objects can yield powerful results.
Oscillation
In colloquial terms, oscillation is the same as vibration, but, in more scientific terms, oscillation can be identified as a type of harmonic motion, typically periodic, in one or more dimensions. All things that oscillate do so either along a more or less straight path, like that of a spring pulled from a position of stable equilibrium; or they oscillate along an arc, like a swing or pendulum.
In the case of the swing or pendulum, stable equilibrium is the point at which the object is hanging straight downward—that is, the position to which gravitation force would take it if no other net forces were acting on the object. For a spring, stable equilibrium lies somewhere between the point at which the spring is stretched to its maximum length and the point at which it is subjected to maximum compression without permanent deformation.
Cycles and Frequency
A cycle of oscillation involves movement from a certain point in a certain direction, then a reversal of direction and a return to the original point. It is simplest to treat a cycle as the movement from a position of stable equilibrium to one of maximum displacement, or the furthest possible point from stable equilibrium.
The amount of time it takes to complete one cycle is called a period, and the number of cycles in one second is the frequency of the oscillation. Frequency is measured in Hertz. Named after nineteenth-century German physicist Heinrich Rudolf Hertz (1857-1894), a single Hertz (Hz)— the term is both singular and plural—is equal to one cycle per second.
Amplitude and Energy
The amplitude of a cycle is the maximum displacement of particles during a single period of oscillation. When an oscillator is at maximum displacement, its potential energy is at a maximum as well. From there, it begins moving toward the position of stable equilibrium, and as it does so, it loses potential energy and gains kinetic energy. Once it reaches the stable equilibrium position, kinetic energy is at a maximum and potential energy at a minimum.
As the oscillating object passes through the position of stable equilibrium, kinetic energy begins to decrease and potential energy increases. By the time it has reached maximum displacement again—this time on the other side of the stable equilibrium position—potential energy is once again at a maximum.
Oscillation in Wave Motion
The particles in a mechanical wave (a wave that moves through a material medium) have potential energy at the crest and trough, and gain kinetic energy as they move between these points. This is just one of many ways in which wave motion can be compared to oscillation. There is one critical difference between oscillation and wave motion: whereas oscillation involves no net movement, but merely movement in place, the harmonic motion of waves carries energy from one place to another. Nonetheless, the analogies than can be made between waves and oscillations are many, and understandably so: oscillation, after all, is an aspect of wave motion.
A periodic wave is one in which a uniform series of crests and troughs follow one after the

A common example of resonance: a parent pushes her child on a swing.
other in regular succession. Two basic types of periodic waves exist, and these are defined by the relationship between the direction of oscillation and the direction of the wave itself. A transverse wave forms a regular up-and-down pattern, in which the oscillation is perpendicular to the direction in which the wave is moving. On the other hand, in a longitudinal wave (of which a sound wave is the best example), oscillation is in the same direction as the wave itself.
Again, the wave itself experiences net movement, but within the wave—one of its defining characteristics, as a matter of fact—are oscillations, which (also by definition) experience no net movement. In a transverse wave, which is usually easier to visualize than a longitudinal wave, the oscillation is from the crest to the trough and back again. At the crest or trough, potential energy is at a maximum, while kinetic energy reaches a maximum at the point of equilibrium between crest and trough. In a longitudinal wave, oscillation is a matter of density fluctuations: the greater the value of these fluctuations, the greater the energy in the wave.
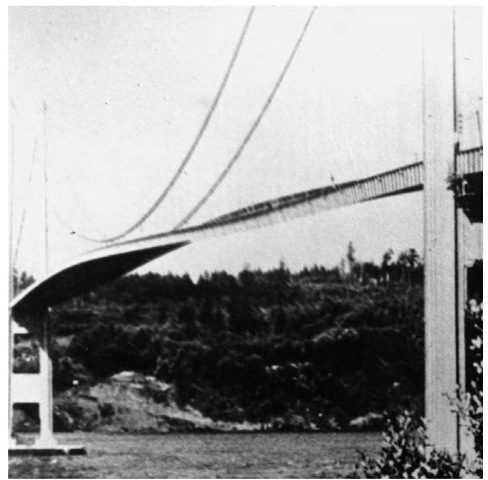
The power of resonance can destroy a bridge. On November 7, 1940, the acclaimed Tacoma Narrows Bridge collapsed due to overwhelming resonance.
Parameters for Describing Harmonic Motion
The maximum value of the pressure change between waves is the amplitude of a longitudinal wave. In fact, waves can be described according to many of the same parameters used for oscillation—frequency, period, amplitude, and so on. The definitions of these terms vary somewhat, depending on whether one is discussing oscillation or wave motion; or, where wave motion is concerned, on whether the subject is a transverse wave or a longitudinal wave.
For the present purposes, however, it is necessary to focus on just a few specifics of harmonic motion. First of all, the type of motion with which we will be concerned is oscillation, and though wave motion will be mentioned, our principal concern is the oscillations within the waves, not the waves themselves. Second, the two parameters of importance in understanding resonance are amplitude and frequency.
Resonance and Energy Transfer
Resonance can be defined as the condition in which force is applied to an oscillator at the point of maximum amplitude. In this way, the motion of the outside force is perfectly matched to that of the oscillator, making possible a transfer of energy.
As its name suggests, resonance is a matter of one object or force “getting in tune with” another object. One literal example of this involves shattering a wine glass by hitting a musical note that is on the same frequency as the natural frequency of the glass. (Natural frequency depends on the size, shape, and composition of the object in question.) Because the frequencies resonate, or are in sync with one another, maximum energy transfer is possible.
The same can be true of soldiers walking across a bridge, or of winds striking the bridge at a resonant frequency—that is, a frequency that matches that of the bridge. In such situations, a large structure may collapse under a force that would not normally destroy it, but the effects of resonance are not always so dramatic. Sometimes resonance can be a simple matter, like pushing a child in a swing in such a way as to ensure that the child gets maximum enjoyment for the effort expended.
REAL-LIFE APPLICATIONS
A Child on a Swing and a Pendulum in a Museum
Suppose a father is pushing his daughter on a swing, so that she glides back and forth through the air. A swing, as noted earlier, is a classic example of an oscillator. When the child gets in the seat, the swing is in a position of stable equilibrium, but as the father pulls her back before releasing her, she is at maximum displacement.
He releases her, and quickly, potential energy becomes kinetic energy as she swings toward the position of stable equilibrium, then up again on the other side. Now the half-cycle is repeated, only in reverse, as she swings backward toward her father. As she reaches the position from which he first pushed her, he again gives her a little push. This push is essential, if she is to keep going. Without friction, she could keep on swinging forever at the same rate at which she begun. But in the real world, the wearing of the swing’s chain against the support along the bar above the swing will eventually bring the swing itself to a halt.
Timing the Push
Therefore, the father pushes her—but in order for his push to be effective, he must apply force at just the right moment. That right moment is the point of greatest amplitude—the point, that is, at which the father’s pushing motion and the motion of the swing are in perfect resonance.
If the father waits until she is already on the downswing before he pushes her, not all the energy of his push will actually be applied to keeping her moving. He will have failed to efficiently add energy to his daughter’s movement on the swing. On the other hand, if he pushes her too soon—that is, while she is on the upswing— he will actually take energy away from her movement.
If his purpose were to bring the swing to a stop, then it would make good sense to push her on the upswing, because this would produce a cycle of smaller amplitude and hence less energy. But if the father’s purpose is to help his daughter keep swinging, then the time to apply energy is at the position of maximum displacement.
It so happens that this is also the position at which the swing’s speed is the slowest. Once it reaches maximum displacement, the swing is about to reverse direction, and, therefore, it stops for a split-second. Once it starts moving again, now in a new direction, both kinetic energy and speed increase until the swing passes through the position of stable equilibrium, where it reaches its highest rate.
The Foucault Pendulum.
Hanging from a ceiling in Washington, D.C.’s Smithsonian Institution is a pendulum 52 ft (15.85 m) long, at the end of which is an iron ball weighing 240 lb (109 kg). Back and forth it swings, and if one sits and watches it long enough, the pendulum appears to move gradually toward the right. Over the course of 24 hours, in fact, it seems to complete a full circuit, moving back to its original orientation.
There is just one thing wrong with this picture: though the pendulum is shifting direction, this does not nearly account for the total change in orientation. At the same time the pendulum is moving, Earth is rotating beneath it, and it is the viewer’s frame of reference that creates the mistaken impression that only the pendulum is rotating. In fact it is oscillating, swinging back and forth from the Smithsonian ceiling, but though it shifts orientation somewhat, the greater component of this shift comes from the movement of the Earth itself.
This particular type of oscillator is known as a Foucault pendulum, after French physicist Jean Bernard Leon Foucault (1819-1868), who in 1851 used just such an instrument to prove that Earth is rotating. Visitors to the Smithsonian, after they get over their initial bewilderment at the fact that the pendulum is not actually rotating, may well have another question: how exactly does the pendulum keep moving?
As indicated earlier, in an ideal situation, a pendulum continues oscillating. But situations on Earth are not ideal: with each swing, the Fou cault pendulum loses energy, due to friction from the air through which it moves. In addition, the cable suspending it from the ceiling is also oscillating slightly, and this, too, contributes to energy loss. Therefore, it is necessary to add energy to the pendulum’s swing.
Surrounding the cable where it attaches to the ceiling is an electromagnet shaped like a donut, and on either side, near the top of the cable, are two iron collars. An electronic device senses when the pendulum reaches maximum amplitude, switching on the electromagnet, which causes the appropriate collar to give the cable a slight jolt. Because the jolt is delivered at the right moment, the resonance is perfect, and energy is restored to the pendulum.
Resonance in Electricity and Electromagnetic Waves
Resonance is a factor in electromagnetism, and in electromagnetic waves, such as those of light or radio. Though much about electricity tends to be rather abstract, the idea of current is fairly easy to understand, because it is more or less analogous to a water current: hence, the less impedance to flow, the stronger the current. Minimal impedance is achieved when the impressed voltage has a certain resonant frequency.
NUCLEAR MAGNETIC RESONANCE
The term “nuclear magnetic resonance” (NMR) is hardly a household world, but thanks to its usefulness in medicine, MRI—short for magnetic resonance imagining—is certainly a well-known term. In fact, MRI is simply the medical application of NMR. The latter is a process in which a rotating magnetic field is produced, causing the nuclei of certain atoms to absorb energy from the field. It is used in a range of areas, from making nuclear measurements to medical imaging, or MRI. In the NMR process, the nucleus of an atom is forced to wobble like a top, and this speed of wobbling is increased by applying a magnetic force that resonates with the frequency of the wobble.
The principles of NMR were first developed in the late 1930s, and by the early 1970s they had been applied to medicine. Thanks to MRI, physicians can make diagnoses without the patient having to undergo either surgery or x rays. When a patient undergoes MRI, he or she is made to lie down inside a large tube-like chamber. A technician then activates a powerful magnetic field that, depending on its position, resonates with the frequencies of specific body tissues. It is thus possible to isolate specific cells and analyze them independently, a process that would be virtually impossible otherwise without employing highly invasive procedures.
LIGHT AND RADIO WAVES
One example of resonance involving visible and invisible light in the electromagnetic spectrum is resonance fluorescence. Fluorescence itself is a process whereby a material absorbs electromagnetic radiation from one source, then re-emits that radiation on a wavelength longer than that of the illuminating radiation. Among its many applications are the fluorescent lights found in many homes and public buildings. Sometimes the emitted radiation has the same wavelength as the absorbed radiation, and this is called resonance fluorescence. Resonance fluorescence is used in laboratories for analyzing phenomena such as the flow of gases in a wind tunnel.
Though most people do not realize that radio waves are part of the electromagnetic spectrum, radio itself is certainly a part of daily life, and, here again, resonance plays a part. Radio waves are relatively large compared to visible light waves, and still larger in comparison to higher-frequency waves, such as those in ultraviolet light or x rays. Because the wavelength of a radio signal is as large as objects in ordinary experience, there can sometimes be conflict if the size of an antenna does not match properly with a radio wave. When the sizes are compatible, this, too, is an example of resonance.
MICROWAVES
Microwaves occupy a part of the electromagnetic spectrum with higher frequencies than those of radio waves. Examples of microwaves include television signals, radar—and of course the microwave oven, which cooks food without applying external heat. Like many other useful products, the microwave oven ultimately arose from military-industrial research, in this case, during World War II. Introduced for home use in 1955, its popularity grew slowly for the first few decades, but in the 1970s and 1980s, microwave use increased dramatically. Today, most American homes have microwaves ovens.
Of course there will always be types of food that cook better in a conventional oven, but the beauty of a microwave is that it makes possible the quick heating and cooking of foods—all without the drying effect of conventional baking. The basis for the microwave oven is the fact that the molecules in all forms of matter are vibrating. By achieving resonant frequency, the oven adds energy—heat—to food. The oven is not equipped in such a way as to detect the frequency of molecular vibration in all possible substances, however; instead, the microwaves resonant with the frequency of a single item found in nearly all types of food: water.
Emitted from a small antenna, the microwaves are directed into the cooking compartment of the oven, and, as they enter, they pass a set of turning metal fan blades. This is the stirrer, which disperses the microwaves uniformly over the surface of the food to be heated. As a microwave strikes a water molecule, resonance causes the molecule to align with the direction of the wave. An oscillating magnetron, a tube that generates radio waves, causes the microwaves to oscillate as well, and this, in turn, compels the water molecules to do the same. Thus, the water molecules are shifting in position several million times a second, and this vibration generates energy that heats the water.
Microwave ovens do not heat food from the inside out: like a conventional oven, they can only cook from the outside in. But so much energy is transferred to the water molecules that conduction does the rest, ensuring relatively uniform heating of the food. Incidentally, the resonance between microwaves and water molecules explains why many materials used in cooking dishes—materials that do not contain water— can be placed in a microwave oven without being melted or burned. Yet metal, though it also contains no water, is unsafe.
Metals have free electrons, which makes them good electrical conductors, and the presence of these free electrons means that the microwaves produce electric currents in the surfaces of metal objects placed in the oven. Depending on the shape of the object, these currents can jump, or arc, between points on the surface, thus producing sparks. On the other hand, the interior of the microwave oven itself is in fact metal, and this is so precisely because microwaves do bounce back and forth off of metal. Because the walls are flat and painted, however, currents do not arc between them.
Resonance of Sound Waves
A highly trained singer can hit a note that causes a wine glass to shatter, but what causes this to happen is not the frequency of the note, per se. In other words, the shattering is not necessarily because of the fact that the note is extremely high; rather, it is due to the phenomenon of resonance. The natural, or resonant, frequency in the wine glass, as with all objects, is determined by its shape and composition. If the singer’s voice (or a note from an instrument) hits the resonant frequency, there will be a transfer of energy, as with the father pushing his daughter on the swing. In this case, however, a full transfer of energy from the voice or musical instrument can overload the glass, causing it to shatter.
Another example of resonance and sound waves is feedback, popularized in the 1960s by rock guitarists such as Jimi Hendrix and Pete Townsend of the Who. When a musician strikes a note on an electric guitar string, the string oscillates, and an electromagnetic device in the guitar converts this oscillation into an electrical pulse that it sends to an amplifier. The amplifier passes this oscillation on to the speaker, but if the frequency of the speaker is the same as that of the vibrations in the guitar, the result is feedback.
Both in scientific terms and in the view of a music fan, feedback adds energy. The feedback from the speaker adds energy to the guitar body, which, in turn, increases the energy in the vibration of the guitar strings and, ultimately, the power of the electrical signal is passed on to the amp. The result is increasing volume, and the feedback thus creates a loop that continues to repeat until the volume drowns out all other notes.
KEY TERMS
Amplitude: The maximum displacement of particles from their normal position during a single period of oscillation.
Cycle: One full repetition of oscillation.
Frequency: For a particle experiencing oscillation, frequency is the number of cycles that take place during one second. Frequency is measured in Hertz.
Harmonic motion: The repeated movement of a particle about a position of equilibrium, or balance.
Hertz: A unit for measuring frequency, named after nineteenth-century German physicist Heinrich Rudolf Hertz (1857-1894). Higher frequencies are expressed in terms of kilohertz (kHz; 103 or 1,000 cycles per second) or megahertz (MHz; 106 or 1 million cycles per second.)
Kinetic energy: The energy that an object possesses due to its motion, as with a sled when sliding down a hill. This is contrasted with potential energy.
Longitudinal wave: A wave in which the movement of vibration is in the same direction as the wave itself. This is contrasted to a transverse wave.
Maximum displacement: For an object in oscillation, maximum displacement is the furthest point from stable equilibrium.
Oscillation: A type of harmonic motion, typically periodic, in one or more dimensions.
Period: The amount of time required for one cycle in oscillating motion.
Periodic motion: Motion that is repeated at regular intervals. These intervals are known as periods.
Periodic wave: A wave in which a uniform series of crests and troughs follow one after the other in regular succession.
Potential energy: The energy that an object possesses due to its position, as, for instance, with a sled at the top of a hill. This is contrasted with kinetic energy.
Resonance: The condition in which force is applied to an object in oscillation at the point of maximum amplitude.
Resonant frequency: A frequency that matches that of an oscillating object.
Stable equilibrium: A position in which, if an object were disturbed, it would tend to return to its original position. For an object in oscillation, stable equilibrium is in the middle of a cycle, between two points of maximum displacement.
Transverse wave: A wave in which the vibration or motion is perpendicular to the direction in which the wave is moving. This is contrasted to a longitudinal wave.
Wave motion: A type of harmonic motion that carries energy from one place to another without actually moving any matter.
How Resonance Can Break a Bridge
The power of resonance goes beyond shattering a glass or torturing eardrums with feedback; it can actually destroy large structures. There is an old folk saying that a cat can destroy a bridge if it walks across it in a certain way. This may or may not be true, but it is certainly conceivable that a group of soldiers marching across a bridge can cause it to crumble, even though it is capable of holding much more than their weight, if the rhythm of their synchronized footsteps resonates with the natural frequency of the bridge. For this reason, officers or sergeants typically order their troops to do something very unmilitary—to march out of step—when crossing a bridge.
The resonance between vibrations produced by wind and those of the structure itself brought down a powerful bridge in 1940, a highly dramatic illustration of physics in action that was captured on both still photographs and film. Located on Puget Sound near Seattle, Washington, the Tacoma Narrows Bridge was, at 2,800 ft (853 m) in length, the third-longest suspension bridge in the world. But on November 7, 1940, it gave way before winds of 42 mi (68 km) per hour.
It was not just the speed of these winds, but the fact that they produced oscillations of resonant frequency, that caused the bridge to twist and, ultimately, to crumble. In those few seconds of battle with the forces of nature, the bridge writhed and buckled until a large segment collapsed into the waters of Puget Sound. Fortunately, no one was killed, and a new, more stable bridge was later built in place of the one that had come to be known as “Galloping Gertie.” The incident led to increased research and progress in understanding of aerodynamics, harmonic motion, and resonance.
