Graphics Reference
In-Depth Information
at the error term E which contains the first, respectively, second derivative as a factor.
Thus we are led to ask if we can find an approximation to the integral that gives the
exact answer on polynomials of degree £ k. We will achieve this if we can find an
approximation whose error term has the (k + 1)st derivative as a factor.
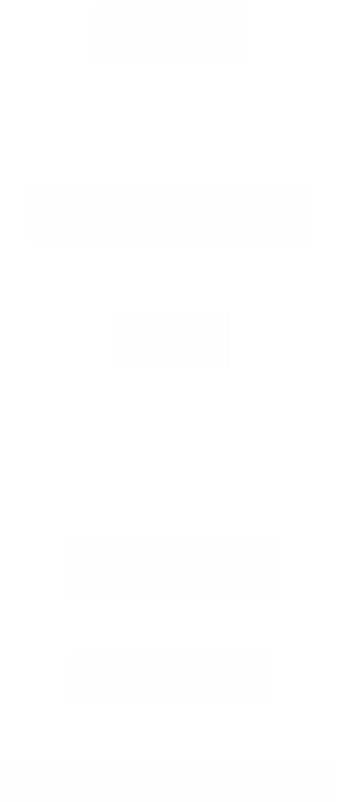
In general, given a function f(x) and a weight function w(x), Gaussian quadrature
gives an approximation for the integral
b
Ú
I
=
f x wx x
() ()
.
(F.7)
a
One uses the inner product on C
r
([a,b]) defined by (F.4b). Thus there are different
flavors of Gaussian quadrature depending on the choice of w(x) in this general
setting.
Legendre-Gauss quadrature
(or simply
Gauss
or
Gaussian quadrature
if one is
only contemplating the integral (F.1)) computes an approximation of this integral (F.7)
when w(x) = 1 and uses the inner product defined by (F.4a).
Chebyshev-Gauss quad-
rature
uses
1
()
=
wx
.
2
1
-
x
The more general type of Gauss quadrature is useful in situations where one is still
trying to approximate an integral of the type (F.1) but the function f(x) has singular-
ities which can be removed by rewriting the integral in the form
()
()
fx
wx
wxdx
b
b
Ú
()
Ú
()
I
=
f x x
=
,
a
a
where
()
()
fx
wx
()
=
Fx
has no singularities.
F.2.5. Theorem.
Let p
i
(x) be the sequence of orthogonal polynomials with respect
to the inner product (F.4b). If x
0
, x
1
,..., x
k
are the zeros of the polynomial p
k+1
(x)
and if we define
k
xx
xx
-
-
0
j
()
=
Lx
(F.8)
ik
,
i
j
j
=π
,
j i
and
b
Ú
() ()
a
=
Lx wx dx
,
(F.9)
i
i k
,
a
then the approximation
()
+
()
+ ◊◊◊+
()
I
=
af x af x
af x
k
00
11
k
k