Graphics Reference
In-Depth Information
for the integral I in (F.7) will be exact whenever f(x) is a polynomial function of degree
£ 2k + 1. Furthermore,
1
22
R
c
k
+
1
(
)
22
k
+
()
II
-=
f
x
k
(
)
k
+
!
2
k
+
1
for some xŒ(a,b), where
b
2
Ú
(
()
)
( )
R
=
p
x
w x du
k
+
1
k
+
1
a
and c
k+1
is the coefficient of the leading term in p
k+1
(x).
Proof.
See [ConD72].
Theorem F.2.5 is one of the basic Gaussian quadrature results. The polynomials
L
i,k
(x) in formula (F.8) are just the
Lagrange basis functions
that are used in Lagrange
interpolation.
Definition.
The zeros of the polynomials in the sequence of orthogonal polynomi-
als with respect to the inner product (F.4b) are called the
Gaussian points
or
zeros
.
The corresponding coefficients a
i
defined by equation (F.9) are called the
Gaussian
weights
.
Now, since the sequences of orthogonal polynomials are well known, so are their
Gaussian zeros and weights, which can be looked up in a table. Therefore, to use
Gaussian quadrature one only has to be able to evaluate the function f(x) at the
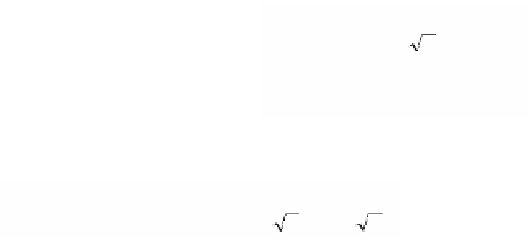
Gaussian zeros. For example, in the case of Legendre-Gauss quadrature with k = 1,
the zeros of
1
2
(
)
2
Px
()
=
31
x
-
2
are
1
3
1
3
x
=-
and
x
=
.
0
1
Also,
1
3
1
3
Ê
Ë
ˆ
¯
x
-
x
--
1
1
Ú
Ú
a
=
dx
=
1
and
a
=
dx
=
1
.
0
1
1
3
1
3
1
3
1
3
1
Ê
Ë
ˆ
¯
1
Ê
Ë
ˆ
¯
-
-
--
Therefore, the two-point Gaussian quadrature approximation is
1
3
1
3
1
Ê
Ë
ˆ
¯
Ê
Ë
ˆ
¯
Ú
fafx afx
ª
()
+
()
=-
f
+
f
.
00
11
1