Environmental Engineering Reference
In-Depth Information
According to Equation (10.187), the semivariogram
is the mirror image of the covariance function. If the
quantity under investigation does not have a finite vari-
ance, then the semivariogram does not have a horizontal
asymptote, and neither a range nor a sill can be
identified.
Kriging on the basis of the intrinsic hypothesis is very
similar to kriging based on the assumption of second-
order stationarity, except that there is no assumption
that the mean and covariance function of the rSF are
known. The estimated value of the rSF,
Z
, is expressed
as the weighted average of measurements,
Z
i
, located at
x
i
, where
From the definition of the semivariogram given in
Equation (10.186)
1
2
1
2
2
2
γ(
x
−
x
)
=
(
Z Z
−
)
=
[(
Z Z
−
)
−
(
Z Z
−
)]
i
j
i
j
i
j
1
2
1
2
=
(
Z Z
−
)
2
+
(
Z Z
−
)
2
i
j
−
(
Z Z Z Z
−
)(
−
)
i
j
=
γ
(
x
−
x
)
+
γ
(
x
−
x
)
−
(
Z Z Z Z
−
)(
−
)
i
j
i
j
(10.192)
Combining Equations (10.191) and (10.192) leads to
the following expression for the estimation error in
terms of the semivariogram
n
∑
λ
1
Z
=
Z
(10.188)
i
i
i
=
n
n
n
n
Assuming that the mean of
Z
(
x
) is uniformly distrib-
uted in space, and that the mean of
∑
∑
∑
∑
Z Z
(
−
)
2
= −
λλγ
(
x
−
x
)
+
λλγ
(
x
−
x
)
Z
is equal to the
i
j
i
j
i
j
i
i
=
1
j
=
1
i
=
1
j
=
1
mean of
Z
(
x
), then
n
n
∑
∑
+
λλ
γ(
x
−
x
)
Z
i
j
j
=
Z
( )
x
=
µ
(10.189)
i
=
1
j
=
1
(10.193)
Taking the expectation of Equation (10.188) yields
utilizing Equation (10.190) and noting that
n
∑
λ
1
Z
=
Z
i
i
n
n
∑
∑
λγ
(
x
−
x
)
=
λγ
(
x
−
x
)
i
=
(10.194)
i
i
j
j
i
=
1
j
=
1
n
∑
i
i
µ
=
λµ
then Equation (10.193) for the estimation error
becomes
=
1
which leads to the following condition on the weights
n
n
n
n
∑
∑
∑
Z Z
∑
=
1
(
−
) =
2
−
λλγ
(
x
−
x
) 2
+
λγ
(
x
−
x
)
λ
i
1
(10.190)
i
j
i
j
i
i
i
=
1
j
=
1
i
=
1
i
=
(10.195)
We now impose the requirement that the mean
square estimation error be minimized. The mean square
error is given by
The constrained minimization of the estimation error
given by Equation (10.195) relative to the station
weights,
λ
i
, and subject to the constraint given by Equa-
tion (10.190) is found using the lagrange multiplier
α
,
in which case the expression to be minimized is
2
2
n
n
n
∑
∑
∑
ˆ
(
Z Z
−
)
2
=
λ
Z Z
−
=
λ
Z
−
λ
Z
i
i
i
i
i
i
=
1
i
=
1
i
=
1
2
n
∑
=
λ
(
Z
−
Z
)
n
1
2
i
i
∑
Z Z
2
(
−
)
−
α
λ
−
1
(10.196)
i
=
1
i
i
=
1
n
n
∑
∑
=
λ
(
Z Z
−
)
λ
(
Z Z
−
)
i
i
j
j
where the estimation error has been divided by 2, and
a minus sign place before the
α
to simplify the subse-
quent expressions. Taking the partial derivatives of this
expression with respect to
λ
i
and
α
lead to the following
system of
n
+ 1 linear equations
i
=
1
j
=
1
n
n
∑
1
=
λλ
(
Z Z Z
−
)(
−
Z
)
i
j
i
j
i
=
1
j
=
(10.191)




























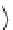
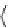


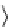



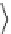




Search WWH ::

Custom Search