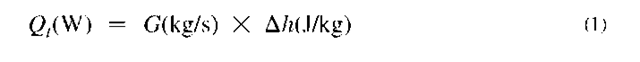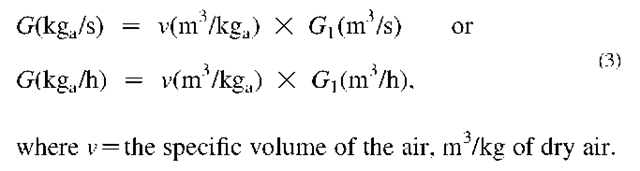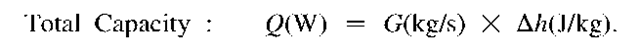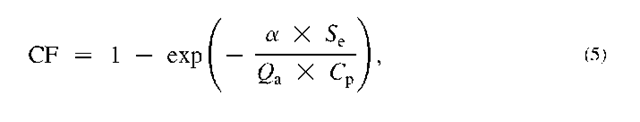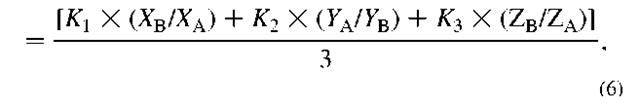Abstract
A vehicle heating, ventilating, and air conditioning system is not just desirable, but it is also necessary standard equipment for vehicles manufactured today. This system provides cab-crew comfort and is important for safety by ensuring demisting of the cab environment and defogging of windows in all kinds of weather. This entry is a sequel to the entry “Mobile HVAC Systems: Physics and Configuration” published in this topic and it provides readers a short synopsis of mobile HVAC system psychrometric fundamentals, components design approaches, and components and system capacity calculations, as well as the future of mobile climate control systems.
ULRINIIIUNO AND HUMLHCLHI UNL
For Definitions and Nomenclature please refer to entry “Mobile HVAC Systems: Physics and Configuration” published in this topic.
INTRODUCTION
This article focuses on an overview of mobile HVAC system psychrometric fundamentals, and provides a short explanation of methods and approaches to system and components testing, capacity calculation, and design. At the end of this entry, prospective systems that take into account environmental impact and current no-idle regulations are presented.
POYCHROMETNIC FUNDAMENTALS, AIR CONDITIONING AND HEATING CAPACITY CALCULATIONS, COILS DESIGN, AND MOBILE HVAC SYSTEM TESTING PROCEDURES
The amount of heat transfer Q (W, kW) that takes place as an air stream passes through a heating or cooling coil is the product of the mass flow rate of the air G (kg/s, sometimes kg/h) and the change in its heat content h (J/kg). During a cooling and dehumidifying process, the air stream undergoes changes in both sensible and latent heat contents. Sensible heat is the heat associated with the change in the air dry-bulb temperature. Latent heat is that amount released by the water vapor as it condenses. Sensible and latent heats are usually combined and expressed as total heat, or enthalpy.[1]
A change in enthalpy includes changes in both sensible and latent heats, but it does not account for the small amount of heat in the condensed water that has left the air stream. For some applications, however, the enthalpy of the condensed water is very small in comparison with the total enthalpy change, and as such, it is neglected for simplicity. When the air stream is heated or cooled without dehumidification, no latent heat transfer takes place. In this case, the heat transfer is said to be all sensible, or total heat transfer is sensible heat transfer.
The air-side heat transfer is balanced on the tube side of the coil, with an equal amount of heat being either absorbed or given up by the fluid flowing through the tubes. Again, the heat transferred is the product of the fluid flow rate G (kg/s or kg/h) and a corresponding change in fluid enthalpy h (J/kg). The fluid enthalpy change may be due to a temperature change only (for single-phase fluids such as water, glycol, oil, etc.), a phase change (evaporating or condensing refrigerants, steam, etc.), or a combination of both.
Psychrometric charts and tables usually present enthalpy, specific volume, and other moist-air properties on a dry-air basis rather than the actual air-water vapor mixture. This convention was adopted because it eliminates having to work with a varied air mass flow rate.
During a dehumidifying process, the total mass flow rate of the air-water vapor mixture changes as the vapor condenses out of the air stream. Because of this vapor loss, the mass of the mixture leaving the process is less than that which entered. The amount of dry air in the air stream remains constant throughout the process, however. Using air properties expressed in terms of this dry-air portion enables us to use a constant air mass flow rate, resulting in much simpler calculations. Then we have total heat transferred
and sensible heat transferred,
where G, an air mass flow rate (kg/s) of dry air; Ah, an enthalpy change of the air (J/kg); Cpa, a specific heat of the air (J/(kga K); and A T, the temperature change of the air.
Flow Rate Conversion to kg/s
For most coil heat transfer applications, flow rates are not given in kg/s (or kg/h). Instead, units are chosen that are consistent with those used in the application of fans, pumps, valves, and other components of the system. Air flow rates are generally given as cubic meters per hour Gj (m3/h) or per second Gi(m3/s). On the tube side, the units are usually in liters per hour or per second (L/h, L/s) for single-phase fluids. For evaporating or condensing refrigerants, the flow rates may be given as kg/s or expressed directly in terms of watts. For proper calculation, it is necessary to convert flow rate in m3/s (m3/h) to kg/s (kg/h) of dry air:
Standard Air
The preceding formula uses the actual air volume flow rate. Most air conditioning equipment, however, is rated on the basis of a standard air flow. Standard air flow is a concept that was established to maintain uniformity in the testing, rating, and application of this equipment. Its use permits relatively simple calculation procedures for determining the performance of coils, fans, and other products. The coil performance ratings always are based on standard air flow rate at sea level (Ta = 20.7°C and Patm = 760 mm Hg) only. By definition, standard air has a density of 1.201385 kg/m3. At sea-level pressure (760 mm Hg) this density corresponds to that of dry air at a temperature of 20.7°C. The corresponding temperature for moist air is higher and depends on the actual moisture content. For practical purposes, 21°C is the generally accepted base temperature.
Conversion of Actual Air Flow to Standard Conditions
Because the performance curves are based on standard air conditions, air-flow rates must be expressed at these conditions as well before the curves are used.
For normal heating and air conditioning applications, the Temperature-Altitude chart may be used when the air flow is given as an actual flow rate at a temperature and/or altitude other than 21 °C and sea-level pressure.
The enthalpy of air also varies with altitude. For cooling and dehumidifying applications at high altitudes, total capacity (watts) calculations should be based on the air enthalpies taken at the altitude in question. Heating and all-sensible cooling calculations are affected to a lesser extent, and conversion to standard conditions is usually the only correction taken.
Airside Heat Transfer (Capacity) Calculations
Usually, the process of mobile HVAC system design includes as a most important stage the evaluation of evaporator and heater coils capacities. There are various methods of this evaluation. Here, we will describe a few of them.
1. Wet-bulb (dewpoint) and dry-bulb temperatures measurement method (using psychrometric chart). Coil samples equipped with devices to measure dry-bulb and wet-bulb temperatures should be mounted on the inlet and outlet (inside the calorimeter enclosure) of the tested device. To obtain hj (enthalpy at the coil inlet), find the intersection of the dry-bulb temperature (from the sampling device) and wet-bulb (from the sampling device) or dewpoint temperature on a psychrometric chart. From this point, follow to the left along the diagonal line to the enthalpy scale. This new point is hj.
To obtain h2 (enthalpy at the coil outlet), find the intersection of the dry-bulb temperature (from the sampling device) and wet-bulb temperature (from the sampling device). From this point, follow to the left, again along the diagonal line to the enthalpy scale. This new point is h2.
The Ah is the difference in enthalpy (hj — h2). Multiply this number by G(kga/s) to obtain the air-side cooling capacity (power) of the tested evaporator Eq. 1. Although not exact, this formula provides reasonable accuracy for the range of application. More accurate calculations using inverse heat transfer methods will be presented below.
2. Condensate collection method. This method involves weighing the condensate collected off the core over a given time interval. To determine enthalpy hi, the approach mentioned in the previous section will be applied. Locate where the dry-bulb temperature (from the sampling device) and the wet-bulb (from sampling device) or dewpoint temperature intersect on a psychro-metric chart. Then follow that point over to the left with a straight edge to find the corresponding enthalpy value (hj) at those conditions. Also, follow the intersection point over to the right to determine how many grains of moisture are going into the core (moisture in).
To determine h2, it is necessary to collect all the water that has condensed off the evaporator over a given time interval (usually 15 min) at the same stable conditions that were used to find hj. Next, weigh the condensate with an accurate scale, and convert the number to grains of moisture per kilogram of dry air (moisture condensed).
Now take the difference between moisture in and moisture condensed; this value is moisture out. Then locate the intersection of grains of moisture per kilogram of dry air (moisture out) and dry-bulb temperature (average air discharge temperature from the thermocouple grid on the core face) on the psychrometric chart. Follow this point over to the left to find the corresponding enthalpy (Tz2).
The Ah is the difference in enthalpy (hj — h2). Multiply this number by G to obtain the air-side cooling capacity (power) of the evaporator.
Tube-Side Heat Transfer Calculations
1. Multiphase fluids.
1. Multiphase fluids.
Evaporator/Condenser Capacity Calculations
To obtain hj (evaporator/condenser inlet side), usually used the refrigerant temperature and pressure measured before expansion device (TXV or OT) for evaporator capacity calculations or at the inlet of the condenser (desuperheated gas) for condenser capacity calculations. By means of the refrigerant Saturation Properties-Temperature table (evaporator) or the refrigerant Superheated Vapor-Constant Pressure Tables (condenser), or any available software, and using measured pressure and temperature, it is easy to find the required enthalpy of refrigerant entering the evaporator/condenser.
To obtain h2 (evaporator outlet, suction side or condenser outlet, subcooling side), use the refrigerant temperature and pressure measured right after evaporator/ condenser outlet. By means of the refrigerant Saturation Properties-Temperature table or any available software, and using measured pressure and temperature, it is easy to find the required enthalpy of refrigerant exiting the evaporator/condenser.
The Ah is the difference in enthalpy (hj — h2). Multiplying this number by G to obtain the refrigerant- (tube-) side cooling capacity (power) of the evaporator/condenser. Be sure to remain consistent with the units.
2. Single-Phase Fluids. The tube-side heat transfer rate must balance the air-side rate. The following formulas are used to determine the capacity by means of the fluid flow rate and the fluid temperature change.
Water Coils (Heaters)
Total Capacity (heat transfer rate):
where N, a fluid flow rate; Cp f., a specific heat of fluid; and AT, a fluid temperature difference between the coil inlet and outlet.
Inverse Heat Transfer Method Approach to HVAC System Coils Design, Identifications, and Capacity Calculations
As already discussed, the main elements of vehicle climate-control systems include the evaporator and condenser coils and the heater core. These items are of primary interest in the area of heat transfer identification or Inverse Heat Transfer Problems (IHTP) because most of the time, the heat transfer for each heat transfer’s stream in the climate-control system is estimated either from empirical equations or from heat balance equations. The estimation of heat transfer by inverse heat transfer methods first and foremost makes it possible to get precise results of heat transfer boundary conditions because these boundary conditions are identified on the basis of real temperature measurements, statistically taking into account the measurement errors and using the stochastic approach to the inverse problem’s solution.[3'5'6] The most considerable advantage of the inverse methods over other approaches to the parameter identification is that the inverse methods do not require explicitly taking into consideration any of the numerous parameters and variables that affect heat transfer inside the HVAC module.
For an understanding of the complexity in evaluating the evaporator capacity or in other calculations of the evaporator’s thermal parameters, let us list the variables that one takes into consideration. These are (1) the area of all evaporator plates, tubes, and fins; (2) temperature differences between the refrigerant and air through the evaporator; (3) thermal conductivity of the evaporator’s material; (4) thickness of the plates and fins; and (5) different types of heat transfer, such as heat convection to the evaporator tubes by refrigerant flow, heat conduction through the evaporator’s walls into the fins, and heat transferring by forced convection to the air stream flowing through the evaporator.
It is nearly impossible (or at best leads to lack of accuracy) to consider or calculate all these parameters, which are just part of the variables that affect the heat transfer in the evaporation process. Attempts to do so, either by the empirical equations or by the heat balance equations, would result in inaccurate or even wrong evaluation. There are many such calculations— i.e., Kurosawa and Noguchi.[2] The authors of this article calculated air-side heat transfer through the evaporator by means of a “contact factor,” which had been determined from the empirical equation
where a, the heat transfer coefficient between the air and the outside evaporator surface; Se, the frontal area of the evaporator; Ga, the air-flow rate; and Cp a., the specific heat of air at constant pressure.
Even from this short example, the calculation of heat transfer evidently is inaccurate to begin with, because Eq. 5 is empirical and, thus, inexact; then the heat transfer coefficient, a, has been identified inaccurately from the imprecise heat balance equations. In addition to the huge number of variables that must be taken into account during the coil design process, as described earlier, one more point should be considered: packaging constraints.
It is obvious that the mobile HVAC system geometrical design and optimization or heat exchanger performance evaluation, based on approximate heat transfer calculations and rough evaluation of heat fluxes (or heat transfer coefficients) by means of simplified heat (energy) balance equations, is unacceptable.
The inverse method approach does not require explicitly taking into account any of the aforementioned variables because the measured temperatures already appear as a product of all parameters and variables that are responsible for the heat transfer process in the HVAC unit. In other words, these measured temperatures implicitly include all heat transfer influences in the HVAC thermal system.
With all the preceding, there is no doubt that the solution of the IHTP for the identification of boundary conditions and the device material’s thermophysical characteristics is the most appropriate approach to getting precise estimates of desired thermal parameters. The concept, idea, and some results of the inverse approach (and utilizing the specifically created method of the Adaptive Iterative Filter), as well as the optimization of mobile HVAC heat exchangers’ geometrical parameters, material selection, and capacity calculation based on this approach, have been presented in references.1-3’5’6-1
Evaporator Core Identification and Inverse Design
As regards the design of air conditioning systems, there is no need to say that it is very expensive and requires significant development work, even after the first prototypes have been built. To overview the complexity of the traditional approach to this design, let us just note that it requires the construction of a very complicated mathematical model of the evaporation process. This mathematical model of heat transfer between the evaporator and the air coming through it should include the following equations:
• Heat transfer from the air to the inner surface of the device
• Heat conduction through the evaporator’s plates and fins
• Forced heat convection with air
• Heat exchange between the air and the device body inside the evaporator
• Heat transfer from the outer evaporator surface to the ambient air
To reduce the cost of design and development, eliminate unnecessary tests, and make the design of the evaporator core more precise, the idea of using inverse methods for identification of thermal parameters between heat exchanger and heat transfer media also has been implemented.[5,6] This approach allows reducing to a minimum the complicated calculation because it performs at the preliminary design stage and, as such, requires minimum time at a minimal cost.
It is apparent that the greatest factor that affects the evaporator performance (heat transfer and capacity) is the coil’s dimensions. These dimensions, as already mentioned, are limited by the packaging constraints, which for vehicle applications are among the most important decisive factors in HVAC system design. The selection of the coil type depends in great part on space availability. The greater the space, the better the evaporator’s overall heat transfer and capacity. On the other hand, the room available for system design relating to the evaporator sometimes appears to be too large for the required heat transfer or cooling capacity. It is clear that in this case, the selection of a smaller evaporator leads to savings, which in turn make the product more competitive. The concept of inverse approach to the optimization of heat exchanger size has been proposed in Moultanovsky 2002.[5] As is evident from previous reasoning, the evaporator’s heat transfer and capacity are functions of a specific evaporator core. The coil’s total heat transfer depends upon the air mass flow through the device and, specifically, through the device’s frontal area. At the same air-flow rate, the heat transfer is defined by the surface area of the evaporator. Actually, the frontal area, as mentioned above, should be separated from the whole apparatus, because the dimensions of this area by and large determine the evaporator performance.
To summarize the influences of heat exchanger dimensions on the device’s heat transfer, one can conclude that the greater the device’s surface area, the better the whole apparatus performs, and vice versa. Very often the evaporator’s required heat transfer can be satisfied by a coil with smaller frontal area than the available space. This statement immediately leads to the approach of the design optimization by means of inverse problem (the so-called inverse design).
An interesting approach to evaporator design optimization that shows considerable promise presented in Moultanovsky 2002 and 2001.[5,6] It was proposed there the evaporator material design and optimization of evaporator dimensions.
Optimization of the evaporator dimensions was based on the following considerations. The total heat transfer of the heat exchanger depends on the air mass flow coming through the device and its frontal area. So as mentioned above, at the same air-flow rate, the heat transfer is defined by the surface area of the evaporator. Based on this statement, Moultanovsky 2001[6] presented the construction of a nomogram that serves as an interconnection between the dimensions or, to be more specific, the size of the frontal area of the evaporator, air-device total heat transfer, and the evaporator’s outer surface temperature. This nomogram enables the designer to choose the preliminary inverse selection (design optimization) of evaporator dimensions, satisfying the total heat transfer value.
As far as the frontal area of the heat exchanger is limited by the space available for the device, this area cannot be varied much to satisfy the requirements for heat transfer. That is why the way for HVAC system to significantly change the heat transfer between the evaporator and the air coming through it, is to produce the item from another material, thereby changing the heat transfer between the device and the surroundings. The material used in evaporator construction is of utmost importance because it greatly affects the thermal conductivity to the evaporation heat transfer process and, thus, the evaporator capacity. Estimation of heat transfer for the evaporator produced from alternative prospective material requires the evaporator’s thermal system mathematical simulation. The latter is impossible without very accurate information about boundary conditions between the existing evaporator and the air. Based on the inverse problem approach to identification of these boundary conditions, Moultanovsky 2002 and 2001[5'6] proposed the construction of similar nomograms that serve as interconnections between the evaporator material (thermal conductivity), air-device heat transfer, and the evaporator’s outer surface temperature (or air-flow rate). These nomograms enable the designer to make the preliminary inverse selection and inverse design of evaporator material. Basically, if given the minimal possible heat flux for the specified size of the evaporator, it is easy to find the evaporator’s material, which provides the required heat transfer.
At the end of the evaporator discussion, it would be fruitful to compare the inverse problem approach of the evaporator capacity calculations with the above-mentioned calorimeter-based calculations. Let us take a quick look at traditional calculations:
1. The air-side heat transfer and capacity are obtained by means of measured air dry-bulb and wet-bulb temperatures, or dry-bulb and dewpoint temperatures, at the inlet and outlet of the evaporator. These measurements and psychrometric charts enable us to calculate the change of enthalpy of the air between the device inlet and outlet.
2. The desired capacity is a result of the multiplication of the air-mass flow rate and the enthalpy change.
3. Within this common approach, the heat of the condensed water that has left the air stream will be neglected for simplicity. To take into account the value of this heat, the humidity ratio has to be included in the calculation process. (The humidity ratio is a ratio of the mass of water vapor to the mass of dry air.) As a result, extra empirical calculations are required.
It is clear that the enthalpy changes and relative humidity values obtained by means of empirical psychro-metric cannot be precise enough to satisfy the growing requirements for accuracy of total heat transfer and/or the capacity of the evaporator.
Capacity Calculations by Means of Inverse Heat Transfer Problem Approach
In fact, the heat transfer and capacity calculated using the IHTP approach make it possible to get the required precise results of desired heat transfer. The heat transfer identification procedure once again is based on accurate measurements of evaporator surface temperature that are already a product of the influence on the device of all three heats: sensible, latent, and condensed water. As a result, the coil’s capacity calculations based on the outcomes of these measurements will include all kinds of heat that affect the performance of the cooling device.[5,6]
Heater Core Identification and Inverse Design
The heat transfer process in the heater core is less sophisticated than that process in evaporator core. The air side of the heater core is much more “controlling” than the coolant side because of the much lower air-side heat transfer coefficient (at least 1.5 times lower). This air-side coefficient greatly affects the transfer of heat from the coolant to the air; therefore, its accurate measurement or identification is of extreme importance. It should be noted once again that this coefficient of heat transfer or corresponding heat flux cannot be determined with sufficient accuracy because of the inaccuracy of traditional methods and, in fact, the incorrect approach to the determination. Following is an explanation of the traditional approach and the methods being used.
The different modes of heat transfer in the heater core consist of forced and natural convection, conduction, and radiation. The hot coolant transfers the heat by convection to the heater tube wall, where it is conducted through the wall into the fins. Then the heat is transferred by forced convection to the air stream flowing through the heater.
It is practically impossible to take into account all the complicated heat transfer in the system under study and to determine the overall heat transfer coefficient by using the heat balance equation between the coolant and air sides and/or by utilizing empirical equations that take into consideration the coolant physical properties and flow. That is why identification of the heat flux or heat transfer coefficient on the basis of IHTP methods with accurate temperature measurement on the surface of the heater core makes it possible to get precise results on the thermal parameters under study.[3,6] The flux identified in such a way is a function of a specific heater core itself. The obtained heat flux (or heat transfer coefficient) is used for the simulation of the heater thermal system following by the calculation of heater capacity and evaluation of the coolant side heater core performance. The heater capacity and performance data are applied to the heater core manufacturing process, as well as to the whole HVAC system performance evaluation. Other applications of the obtained boundary conditions are the same as explained above for the evaporator design, such as heater material inverse design and coil inverse design optimization.
New Approach to Mobile HVAC Components Evaluation
During the design phase of a mobile HVAC unit, many factors play a key role in the evaluation of system performance. The most decisive factors, however, are the actual performance of each component. These components include the evaporator, heater, and blower package. Any one of these components can have a dramatic impact on overall system performance. Traditional performance evaluation techniques do not allow for the all-around comparison of each component.
The special formulas and, respectively, special numerical values have been created (see Moultanovsky and Hermann 2001)[4] for each component comparison problem. The numerical value for component comparison is a Common Comparison Coefficient (CCC).
The traditional practice in evaluating blower and heat exchanger performance is to compare components based on air flow at a given backpressure or capacity at a given air flow. If the capacity of one of the coils is better than the capacity of another but pressure drop is worse, for example, the dilemma of which parameter is most significant for performance evaluation arises. Moreover, these two parameters are not the only factors that need to be integrated into the comparison. This list includes, but is not limited to, indoor and outdoor air temperature difference, both air and liquid-/refrigerant-side pressure drops, etc.
The same is true for blower comparisons. At least three parameters should be taken into consideration: airflow, rpm, and power consumption. Very often, they contradict one another, and as a result, the same dilemma arises.
In summary, each of the above-mentioned factors has an impact on performance independently. If any potential factor is left unchecked until final validation and testing, however, a significant failure of the HVAC system could go unchecked until the defects are correctable only by expensive and unnecessary redesign and tooling changes. Moultanovsky 2001[4] proposes a method of comparison through the use of weighting coefficients based on the emphasis of importance to the customer, designer, application, or manufacturer. This method of calculating performance will ensure that no factors or parameters have been overlooked. Common Comparison Coefficient calculations may have as many factors or parameters in the calculation as necessary to optimize the evaluation of overall performance. Overlooking these factors could become costly if they are not included in a performance evaluations. Common Comparison Coefficient is the numerical value that takes into account all compatible parameters produced during the testing of a product or a component.
The following calculation presents a comparison between component A and component B when component A is considered to be the baseline and its performance is accepted as 1 or 100%. It compares component A and component B using 3 different factors/parameters: Factor 1-X, Factor 2-Y, and Factor 3-Z.
It is clear that some parameters/factors are considered to have a positive impact on performance (such as capacity on heat exchangers or air flow on blower packages), whereas other parameters/factors will inversely affect performance (pressure drop through coils or power consumption on blowers). Let us assume that parameters X and Z are considered to have a positive impact on performance, whereas Y will be negative. The CCC can be calculated by the following formula (component A is considered to be the baseline):
CCC
where K\, K2, and K3 are the weight coefficients for each parameter according to importance. For more details on using this approach, see Moultanovsky and Herrmann 2001.[4]
MOBILE HVAC SYSTEM INNOVATIONS AND THE FUTURE
The next generation of air conditioning and heating systems is particularly important to the commercial success of electric, hybrid, fuel-cell, and other low-emission vehicles, which can capture market share only if they are equipped with good-performance, highly energy-efficient, and reliable cooling and heating systems.[7] Another, very important issue that significantly influences the future of mobile HVAC systems is a vehicle’s anti-idling rules and regulations. These regulations have an effect on many categories of vehicles, including trucks, motor coaches, transit buses, and shuttle and school buses. An average truck idles approximately 2400 h/yr, burning about 2400 gal of fuel during that period. Assuming that 1 gal of fuel costs $3, the cost of 1 truck idling its engine is about $7200/yr. Total idling time for all Class 8 trucks in the United States is about 1 billion h/yr, which accordingly results in 1 billion gal of diesel/yr or $3 billion. If the trucks are not idling, the engine regular service intervals will be significantly increased, and engine oil breakdown will be reduced. Major savings will also be realized as the time between scheduled major preventive-maintenance tear-downs will be increased. Last but not least is a pollution and greenhouse effect.
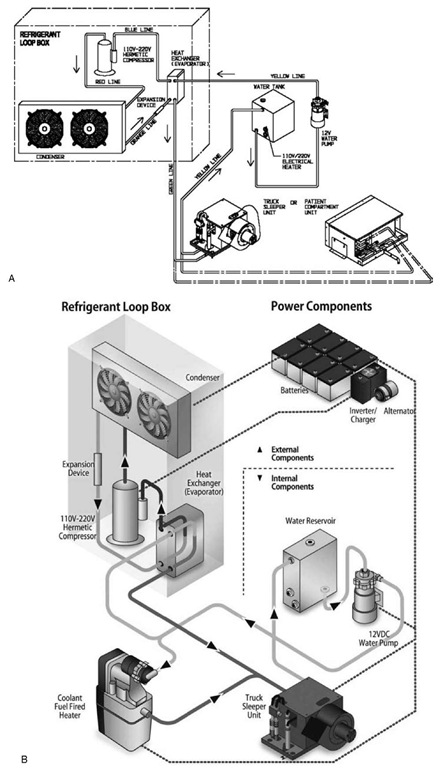
Fig. 1 Electrified, self-contained, secondary loop, hermetically sealed mobile HVAC system. (A) Schematic with electrical heating elements. (B) Complete system diagram with coolant fuel heater, internal and external components, and power package.
Fig. 2 Air-to-air fuel-fired heater.
Strategies for reducing greenhouse-gas emissions and decreasing or eliminating idling is an electrical HVAC systems and, where feasible, shifting to hermetically sealed design.
Today’s automotive AC systems are designed to use less refrigerant. To protect environment the US Environmental Protection Agency encourages all owners of the vehicles manufactured before 1995 that used HVAC systems with CFC-12 refrigerant to retrofit these systems to HFC-134a or other approved alternative refrigerants. New refrigerants that are being considered for future mobile AC systems are CO2 (R744) and slightly flammable HFC-152a (to use with secondary loop systems).[7]
A very promising approach to mobile HVAC systems that provides a complete solution for the entire industry is a secondary loop system. This methodology makes it possible to build a self-contained, engine-driven AC system as well as an electrified, hermetically sealed system that complies with all no-idle regulations. An excellent example of such a system has been brought to the market by ACC Climate Control, Inc., Elkhart, Indiana. Fig. 1 presents a schematic (A) and complete diagram (B) of this secondary-loop, electrified system.
This revolutionary (for mobile HVAC systems) technology—a secondary-loop, hermetic system— includes 1 box containing a condenser coil, a hermetic electrical compressor, and a heat exchanger. Another part of this system consists an antifreeze reservoir with electrical water heaters (Fig. 1A) and an electrical water pump. Alternative source of the heat is a coolant fuel fired heater (Fig. 1B). Figure 1B represents the complete no-idle mobile HVAC system diagram with internal components (installed inside the vehicle), external parts (mounted on the frame, outside of very expensive inside compartment) as well as power package includes battery pack, inverter/charger (allows to use shore power when its available), and alternator. The beauty of this system is the installation connection process. When the components are installed on the vehicle and wiring is complete, heater hoses need to be spliced into the heater lines running from the engine to the auxiliary heater coil, using vacuum or electric water-flow valves. This system allows installing at low cost an extra heat exchanger (or heat exchangers) in the coolant line if additional air conditioning or heating points are required. This will eliminate the necessity for a less-efficient air ducting system. The system can be powered from a 110-VAC or 220-V power source, either from shore power or an auxiliary power unit on the vehicle, or from a 12/24/48-VDC battery package (as mentioned above, last system is designed for dual power: battery package and shore power). When the engine is shut down, the system operates at the same comfort level as when the vehicle (truck, bus, or specialty/ emergency vehicle) engine was running. The secondary-loop approach eliminates refrigerant leaks and enhances high reliability. The system is environmentally friendly and compliant with all no-idle laws and regulations.
A new approach to satisfying vehicle heating requirements is the fuel-fired heaters brought to the mobile market by Espar, ACC Climate Control, and Webasto (Fig. 2).
These diesel fuel-powered heaters can serve as primary auxiliary heaters and ventilators while driving or as heaters without the engine idling for extended or overnight stops. The heaters are compact, lightweight, and quiet when operating. The heaters’ efficient operation reduces both fuel costs and air pollution, and they comply with all no-idle regulations.
All future mobile HVAC systems, as well as systems under development, integrate cooling, heating, defrosting, demisting/defogging, air filtering, and humidity control. They increase driver alertness and visibility (demisting/ defogging windows), as well as passengers’ security. The success of air conditioning systems requires customer acceptance (cooling performance and reliability), operational and service safety, environmental performance, and serviceability. New mobile HVAC systems must anticipate future industry technology—such as higher engine efficiency (less waste heat and electrification) and electric, hybrid, fuel-cell, and low-emission vehicles— while being high-efficiency and satisfactory-performance devices. All vehicles benefit from efficient cooling and heating.[7]