Forces and dynamics - Physical Processes in Earth and Environmental Sciences - page 65

have come across the concept of a second differential in
this topic, the first was for acceleration, as rate of change of
velocity with time. Luckily this particularly second differ-
ential can be just as easily interpreted physically; it is the
rate of change of velocity gradient with distance. In other
words it is a spatial acceleration in the sense discussed
in Section 3.2. So we have just derived Newton's
Second Law again, force equals mass times acceleration,
but this time in a physical way as the action of viscosity
upon a gradient in velocity across unit area, that is,
F viscous

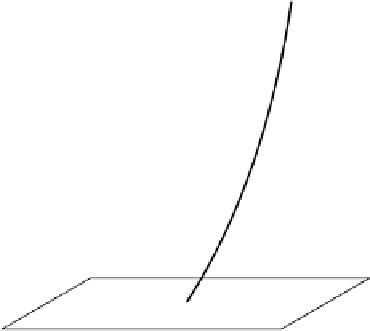
In a boundary layer where the gradient
of velocity changes vertically there
exists a gradient of viscous stress and
thus a net force, positive for the case
illustrated

( d u/ d z) z 2

z 2

(d u/ d z ) z 1 > (d u/ d z ) z 2

|d 2 u /d z 2 |.

t zx

3.10.3 The sign of the net force

dz

− t zx

But one thing is missing from our discussion above - the
sign of the net force. Thinking physically again we would
expect the viscosity to be opposing the rate of change of
fluid motion, giving a negative sign to the term, that is,
F viscous

( d u/ d z) z 1

z 1

Velocity, u

d 2 u /d z 2 ]. For the particular case of the
boundary layer we need to look again at the nature of veloc-
ity change; the velocity is decreasing less rapidly per given
vertical axis increment the further away from the boundary
we get. We will play a simple mathematical trick with this
property of the boundary layer later in this topic; for the
moment we will not specify the exact nature of the change.
Now, since the rate of change is negative, the net viscous
force acting must be overall positive in all such cases.

[

Fig. 3.45 To show definitions of velocity gradients and viscous shear
stresses in a boundary layer whose velocity is changing in space
across an imaginary infinitesimal shear plane, z . Such boundary

layers are very common in the natural world and the resulting net
viscous force reflects the mathematical function of a second
differential coefficient of velocity with respect to height, that is,
F viscous d zx /d z - d 2 u /d z 2 .

3.11 Turbulent force

Turbulent flows of wind and water dominate Earth's
surface. Much of the practical necessity for understanding
turbulence originally came from the fields of hydraulic
engineering and aeronautics. It is perhaps no coincidence
that “modern” fluid dynamical analysis of turbulence
started around the date of Homo sapien s' first few
uncertain attempts at controlled flight. Eighty years later
photographs of turbulent atmospheric flows on Earth
were taken from the Moon, and using radar we can now
image turbulent Venusian and Martian dust storms.

The wind may be steady when averaged over many min-
utes, but varies in velocity on a timescale of a few seconds
to tens of seconds; thus a slower period is followed by a
period of acceleration to a stronger wind, the wind
declines and the process starts over again. This is the essen-
tial nature of turbulence; seemingly irregular variations in
flow velocity over time (Figs 3.46 and 3.47). If we investi-
gate a scenario where we can keep the overall discharge
of flow constant, such as in a laboratory channel, then
we still have the fluctuating velocity but within a flow that
is overall steady in the mean . Insertion of a sensitive
flow-measuring device into such a turbulent flow for a
period of time thus results in a fluctuating record of fluid
velocity but with a statistical mean over time. By way of
contrast, in steady laminar flow any local velocity is always
constant.

3.11.1 Steady in the mean

We know about the intensity of turbulence from experi-
ence, like the gusty buffeting inflicted by a strong wind.