Forces and dynamics - Physical Processes in Earth and Environmental Sciences - page 37

… a lithosphere plate
mean density 3,000 kg m -3
mean thickness 75 km
area 4.0 . 10 6 km 2
velocity 50 mm year -1

… a moving, floating iceberg
mean density 1,000 kg m -3
mean thickness 1,000 m
area 100 km 2
velocity 0.1 ms -1

… is p = c .1.4 . 10 12 kg m s -1

… is p = 1.0 . 10 12 kg m s -1

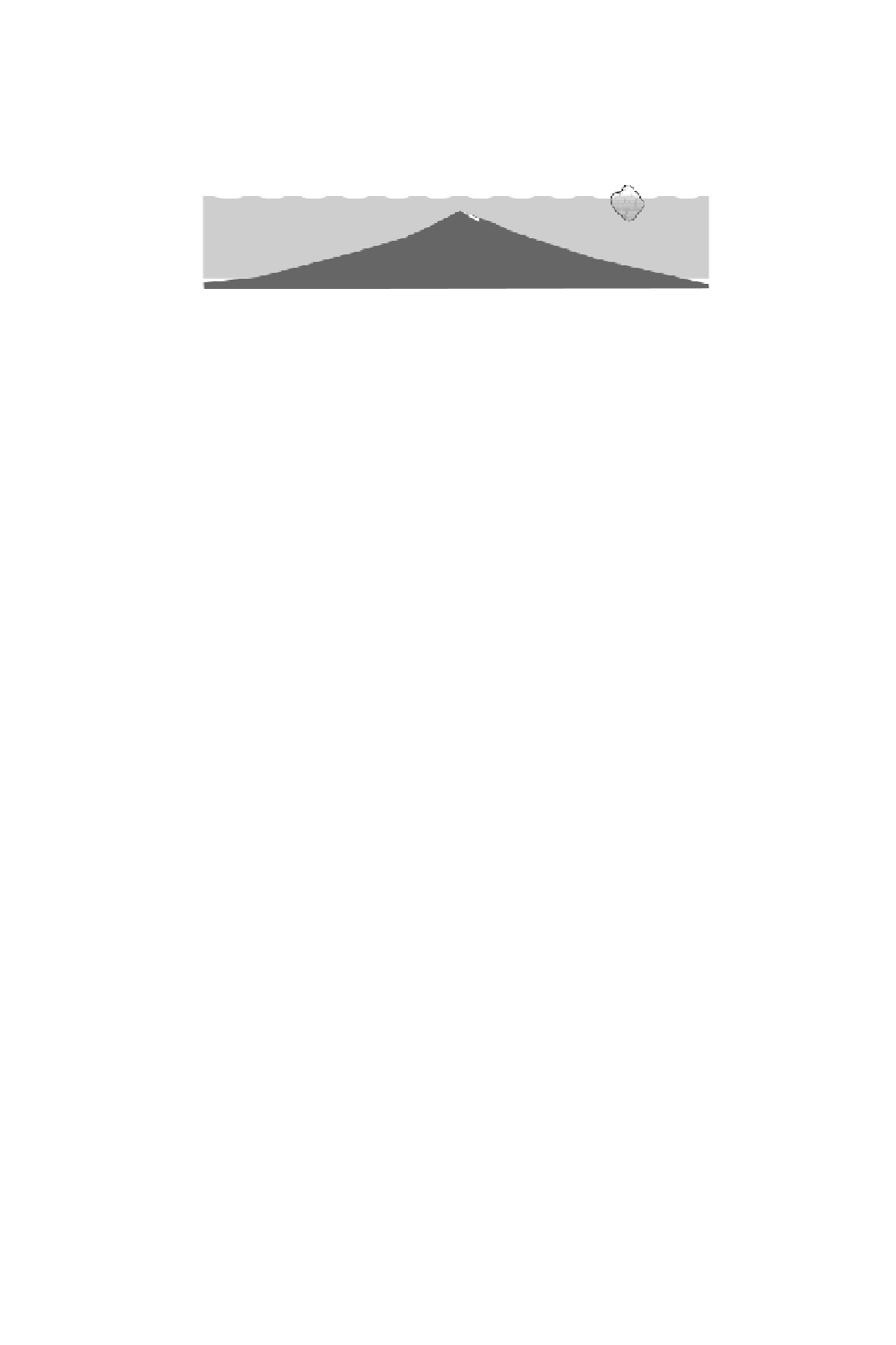
Ocean

Mid-ocean
ridge

Plate

Plate

Fig. 3.3 The momentum of the iceberg (not drawn to scale) is of the same order of magnitude as that of the plate.

3.1.2 Angular momentum

… Earth´s angular momentum, L = Iv , is

v

In considering the momentum of rotating solid objects,
such as planets, sand grains, or figure skaters, it is necessary
to determine the angular momentum (Fig. 3.4) arising
from the rotational motion, rather than the linear momen-
tum of the mass. The momentum is thus considered as
that arising about a rotational axis. The angular momen-
tum, L , is given by the product of rotational inertia (often
called moment of inertia ) about its rotation axis, I , and its
vectorial angular velocity,

r

v = 7.3 10 -5 rad s -1
m = 6

10 24 kg
r = 6.4 10 6 m
I = 10 10 37 kg m 2
L = 7 10 33 kg m 2 s -1

m

. Notice that the
mass term relevant to the determination of linear momen-
tum is here replaced by a rather unfamiliar quantity,
rotational inertia. This is a subtle concept arising from
the notion of rotational kinetic energy (Section 3.3) and
the fact that in rigid body rotation each small element of
mass, m , of a solid can be considered to have its own angu-
lar velocity of rotation,

. Thus L I

… spinning sand grain´s angular momentum, L = Iv , is

= 50 rad s -1
m = 1.15 10 -5 kg
r = 1 10 -3 m
I = 4.6 10 -12 kg m 2
L = 2.3 10 -10 kg m 2 s -1

v

, and therefore kinetic energy,
about any rotation axis. All motion is considered about
this rotation axis: every small element has its own defined
measurable perpendicular distance, R , from this axis and a
characteristic speed of R

Kinetic energy of rotation

E k = 0.5 mR 2

2 = 0.5 2

v I v

. The rotational kinetic energy of
the small element is also related to its angular momentum.
The rotational inertia and angular momentum of the
whole body must be taken as the sum or integral
(appendix) of each small element. Particular regular
shapes have specific integral solutions, for example, the
rotational inertia of a uniform density solid sphere of
radius, r , is given by 2/5 mr 2 . Making use of this expres-
sion we can easily calculate the approximate rotational
inertia and angular momentum of Earth (ignoring its
internal density layering) or of a spinning sand grain
(Fig. 3.4).

Fig. 3.4 Angular momentum. The rotational inertia, I , of a solid
sphere is 2/5 mr 2 .

3.1.3 Dynamic significance of momentum and inertia

The big clue concerning the significance of momentum can
be approached simply from first principles. We have seen that
mass gives us a measure of the quantity of matter present in
a solid or fluid; this helps determine an object's inertia , its
tendency to carry on in the same line of motion or to resist