Geoscience Reference
In-Depth Information
bifurcating wave will be different for the portion of the
transition curve on either side of these points. At such
points, there are two complex conjugate pairs of eigen-
values with zero real part, while all other eigenvalues have
negative real part, i.e., two rotating waves of different wave
number bifurcate simultaneously as parameters are var-
ied through this point. These critical values define the
locations of mode interaction points (also called double
Hopf bifurcation points). The nonlinear interaction of the
two bifurcating waves will produce interesting dynamics
that will be observed as parameters are varied near these
critical points.
Suppose that the mode interaction points have been iso-
lated at the critical parameter values
=
0
and
T
=
T
0
so that for
near
0
and
T
near
T
0
there are
eigenvalues
(4, 3)
m
=5
m
=6
m
=7
(5, 4)
(6, 5)
10
0
(7, 6)
m
=3
m
=4
10
-1
(8, 7)
(7, 8)
(6, 7)
10
-2
Theoretical transition curve
Theoretical critical wave number transitions
Experimental transition curve
Experimental critical wave number transitions
Neutral stability curves
(5, 6)
10
-3
λ
1
=
μ
1
+
iω
1
,
λ
1
,
λ
2
=
μ
2
+
iω
2
,
λ
2
,
(2.13)
10
6
10
7
where
μ
j
=
μ
j
(
,
T)
and
μ
j
(
0
,
T
0
)
=0for
j
=1,2,
i.e., at
=
0
and
T
=
T
0
, there are two complex
conjugate pairs of eigenvalues with zero real part. Also,
assume that all the other eigenvalues have negative real
parts.
The eigenfunctions corresponding to the above eigen-
values are
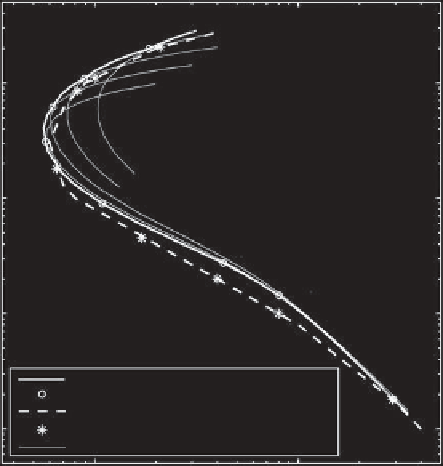
Taylor number
Figure 2.1.
Primary transition curve with neutral stability
curves for moderate Prandtl number, Pr
7.1 (water). Repro-
duced following
Lewis and Nagata
[2003] with the numerical
linear stability analysis results of
Lewis and Nagata
[2003] and
the experimental results of
Fein
[1973].
≡
1
,
1
,
2
,
2
,
The linear stability analysis only indicates the stabil-
ity of the axisymmetric solution and does not determine
to what the perturbations from an unstable solution will
equilibrate. The nonlinear analysis discussed in the next
section may provide some insight into this.
where they have the form
j
=
u
m
j
(r
,
z)
,
T
m
j
(r
,
z)
e
im
j
ϕ
,
˜
with
m
j
(
j
=1,2,
m
1
=
m
2
) being the azimuthal wave
number corresponding to
j
. The adjoint eigenfunctions
corresponding to the
j
are denoted by
j
, where the
j
are found from the adjoint eigenvalue problem.
We write
U
as
2.4. NONLINEAR ANALYSIS
Due to the rotational symmetry of the annulus, all
eigenvalues for nonzero wave numbers will come in com-
plex conjugate pairs (see, e.g.,
Hennessy and Lewis
[2012]).
Except at isolated points along the transition curve, there
is a single complex conjugate pair of eigenvalues with zero
real part, and thus we expect that the transition corre-
sponds to a Hopf bifurcation. Consequently, we expect
that a periodic orbit is born at the transition. Whether this
periodic orbit is stable or unstable can be determined by
the nonlinear analysis. Due to the form of the eigenfunc-
tions, we expect that this periodic orbit corresponds to a
rotating wave (i.e., a baroclinic wave) with azimuthal wave
number corresponding to that of the eigenfunction of the
eigenvalue with zero real part at the transition.
At isolated points along the transition curve, specifically
at the intersection of the neutral stability curves of dif-
ferent wave number, there is what we call a wave number
transition. That is, we expect that the wave number of the
U
=
z
1
1
+
z
1
1
+
z
2
2
+
z
2
2
+
,
(2.14)
E
s
,the
center eigenspace
E
c
the span of the eigenfunctions cor-
responding to the eigenvalues with zero real parts when
=
0
and
T
=
T
0
and the stable eigenspace
E
s
the
span of all the other eigenfunctions, which are the eigen-
functions that correspond to eigenvalues with negative
real parts.
Given certain technical assumptions on (2.10), we can
invoke the center manifold theorem, which enables us
to determine the dynamics of the full partial differential
model equations (PDEs) close to the bifurcation point
from a low-order ordinary differential equation (ODE)
[
Henry
, 1981;
Lewis and Nagata
, 2003]. The theorem states
that there exists an exponentially attracting manifold that
is tangent to the center eigenspace
E
c
, and thus, close to
E
c
and
where
z
1
1
+
z
1
1
+
z
2
2
+
z
2
2
∈
∈