Geoscience Reference
In-Depth Information
3.3.1
The dynamical balance
For a frictionless ocean (t
x
¼
t
y
¼
@
@
¼ @
@
¼
0) in a steady state of motion (
u/
t
v/
t
0)
with no external forces acting (F
x
¼
F
y
¼
0), the linearised momentum
equations
(3.13)
reduce to a balance between the pressure gradient and the Coriolis force:
0
@
0
@
1
p
1
p
fu
¼
y
;
fv
¼
ð
3
:
16
Þ
@
@
x
where r
0
is the average density introduced in the last section. These are the equations
of geostrophic flow. Squaring and adding the two relations gives:
!
!
2
þ
@
2
2
1
@
p
p
1
@
p
f
2
u
2
v
2
f
2
C
g
¼
ð
þ
Þ¼
¼
ð
:
Þ
3
17
0
@
x
@
y
2
@
n
which relates the current speed C
g
to the magnitude of the pressure gradient
@
p/
@
n,i.e.:
0
f
@
1
p
C
g
¼
n
:
ð
3
:
18
Þ
@
The derivative
n here is taken in a direction n normal to the isobars which is the
direction of maximum gradient, illustrated in
Fig. 3.4
. In much of the rest of this
discussion we will use n as our general horizontal axis. The direction of the flow is at
an angle c which can be found by dividing the x and y
equations (3.16)
to give:
@
p/
@
:
v
u
¼
@
p
=@
x
tan c
¼
ð
3
:
19
Þ
@
p
=@
y
This tells us something very important about the direction of a geostrophic flow.
The direction of an isobar in the horizontal plane (
@
y/
@
x)
p
is set by the condition that
Figure 3.4
Geometry of isobars
in the horizontal plane.
p
1
C
g
v
y
n
p
2
u
p
3
p
4
y
y
x










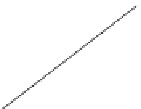



















Search WWH ::

Custom Search