Graphics Reference
In-Depth Information
C
3
1
4
b
2
5
b
B
′
A
′
P
3
4
b
3
5
a
C
′
1
2
A
B
2
3
c
1
3
c
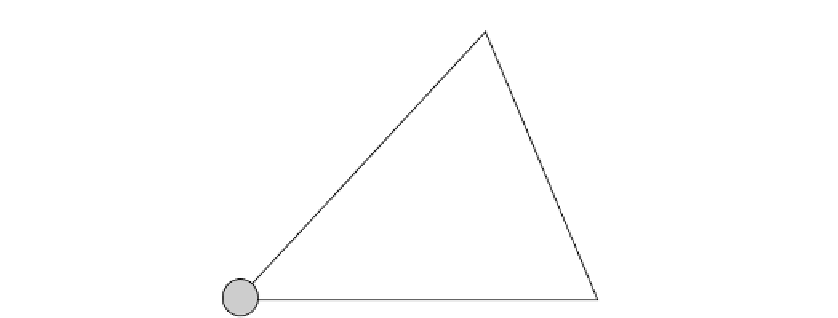
Fig. 11.9.
How the masses determine the positions of
A
,B
and
C
.
Now let's consider another example where
m
A
=1
,m
B
=2and
m
C
=3,
as shown in Figure 11.9.
For a balance condition
A
must be
3
2
5
a
from
B
and
5
a
from
C
. Equally,
B
must be
1
3
4
b
from
A
. Similarly,
C
must be
2
4
b
from
C
and
3
c
from
A
and
1
3
c
from
B
.
Ceva's Theorem confirms that the lines
AA
,BB
and
CC
are concurrent
at
P
, because
2
3
1
AC
C
B
·
BA
A
C
·
CB
B
A
3
c
1
5
a
2
4
b
3
=
3
c
·
5
a
·
4
b
=1
Arbitrarily select
C
C
.At
C
we have an effective mass of 3 (1 + 2) and 3 at
C
,
which means that for a balance condition
P
is mid-way along
C
C
. Similarly,
P
is
3
along
B
B
.
Once more, if we use (11.8) in this scenario we obtain
1
6
along
A
A
and
1
P
=
1
6
A
+
1
3
B
+
1
2
C
Note that the multipliers of
A
,
B
and
C
are identical to the proportions of
P
along
A
A, B
B
and
C
C
. Let's prove why this is so.
Figure 11.10 shows three masses with the triangle's sides divided into their
various proportions to derive
P
.
On the line
A
A
we have
m
A
at
A
and effectively
m
B
+
m
C
at
A
,which
means that
P
divides
A
A
in the ratio
m
A
m
A
+
m
B
+
m
C
m
B
+
m
C
m
A
+
m
B
+
m
C
.
On the line
B
B
we have
m
B
at
B
and effectively
m
A
+
m
C
at
B
,which
means that
:
P
divides
B
B
in the ratio
m
B
m
A
+
m
B
+
m
C
m
A
+
m
C
m
A
+
m
B
+
m
C
.
Similarly, on the line
C
C
we have
m
C
at
C
and effectively
m
A
+
m
B
at
C
, which means that
:
P
divides
C
C
in the ratio
m
C
m
A
+
m
B
+
m
C
m
A
+
m
B
:
m
A
+
m
B
+
m
C
.