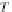Geoscience Reference
In-Depth Information
therefore yield unsatisfactory results. In recent decades, new techniques for
nonlinear data analysis derived from chaos theory have become increasingly
popular. Such methods have been employed to describe nonlinear behavior
by, for example, dei ning the scaling laws and fractal dimensions of natural
processes (Turcotte 1997, Kantz and Schreiber 1997). However, most
methods of nonlinear data analysis require either long or stationary data
series and these requirements are rarely satisi ed in the earth sciences. While
most nonlinear techniques work well on synthetic data, these methods are
unable to describe nonlinear behavior in real data.
During the last decades,
recurrence plots
have become very popular
in science and engineering as a new method of nonlinear data analysis
(Eckmann 1987, Marwan 2007). Recurrence is a fundamental property of
dissipative dynamical systems. Although small disturbances in such systems
can cause exponential divergence in their states, at er some time the systems
will return to a state that is close to a former state and then pass again
through a similar evolution. Recurrence plots allow such recurrent behavior
of dynamical systems to be visually portrayed. h e method is now widely
accepted as a useful tool for the nonlinear analysis of short and nonstationary
data sets.
Phase Space Portrait
h e starting point for most nonlinear data analyses is the construction of a
phase space portrait for a system. h e state of a system can be described by its
state variables
x
1
(
t
),
x
2
(
t
), …,
x
d
(
t
). As an example, suppose the two variables
temperature
and
pressure
are used to describe the thermodynamic state of
the earth's mantle as a complex system. h e
d
state variables at time
t
form
a vector in a
d
-dimensional space, which is known as the phase space. h e
state of a system typically changes with time and the vector in the phase space
therefore describes a trajectory representing the temporal evolution (i.e., the
dynamics) of the system. h e trajectory provides essential information on
the dynamics of the system, such as whether systems are periodic or chaotic.
In many applications the observation of a natural process does not yield
all possible state variables, either because they are not known or because
they cannot be measured. However, due to coupling between the system's
components, we can reconstruct a phase space trajectory from a single
observation
u
i
:
where
m
is the embedding dimension and ˄ is the time delay (index based;