Cryptography Reference
In-Depth Information
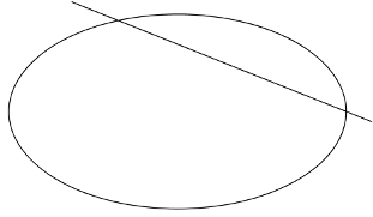
u,v
C
L
u
0
,v
0
Figure 2.6
Since
t
= 0 corresponds to (
u
0
,v
0
), we factor out
t
and obtain
2
au
0
+2
bv
0
m
a
+
bm
2
t
=
−
.
Therefore,
2
amu
0
+2
bv
0
m
2
a
+
bm
2
2
au
0
+2
bv
0
m
a
+
bm
2
u
=
u
0
−
,
v
=
v
0
−
.
We make the convention that
m
=
∞
yields (
u
0
, −v
0
),whichiswhatweget
if we are working with real numbers and let
m →∞
. Also, possibly the
denominator
a
+
bm
2
vanishes, in which case we get points “at infinity” in the
uv
-projective plane (see Exercise 2.14).
Notethatif(
u, v
)isanypointon
C
with coordinates in
K
, then the slope
m
of the line through (
u, v
)and
P
is in
K
(or is infinite). We have there-
fore obtained a bijection, modulo a few technicalities, between values of
m
(including
)andpointson
C
(including points at infinity). The main point
is that we have obtained a parameterization of the points on
C
. A similar
procedure works for any conic section containing a point with coordinates in
K
.
Which value of
m
corresponds to the original point (
u
0
,v
0
)? Let
m
be the
slope of the tangent line at (
u
0
,v
0
). The second point of intersection of the
tangent line with the curve is again the point (
u
0
,v
0
), so this slope is the
desired value of
m
.Thevalue
m
= 0 yields the point (
−u
0
,v
0
). This can be
seen from the formulas, or from the fact that the line through (
−u
0
,v
0
)and
(
u
0
,v
0
) has slope 0.
We now want to intersect
C
, regarded as a “cylinder” in
uvw
-space, with
the surface
cu
2
+
dw
2
=
f
. Substitute the expression just obtained for
u
to
obtain
∞
c
u
0
−
2
2
au
0
+2
bv
0
m
a
+
bm
2
dw
2
=
f
−
.
Search WWH ::

Custom Search