Biomedical Engineering Reference
In-Depth Information
the flow geometry properly in order to use the Nusselt number
to calculate a value for the convective heat transfer coefficient
h
using Equation 1.35.
The Nusselt number is generally determined for a particular
convection process as a function of the interface geometry, flow
properties of the fluid at the interface, and thermodynamic state
of the fluid. These properties are in turn represented by dimen-
sionless ratios defined as the Reynolds, Prandtl, and Grashoff
numbers, as described below.
he
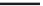
Reynolds number
is defined by the dimensionless ratio
diffusivity. It represents a measure of the relative effectiveness
of diffusive momentum and heat transport in the velocity and
thermal boundary layers. It provides an indication of the relative
thickness of these two boundary layers in a convective system.
As a general guideline for the broad range of Prandtl number
values that may be encountered: for vapors,
Pr
v
≈ δ/δ
T
≈ 1; for
liquid hydrocarbons such as oils, Pr
hc
≈ δ/δ
T
>> 1; and for liquid
metals,
Pr
lm
≈ δ/δ
T
<< 1.
For forced convection processes the Nusselt number is calcu-
lated from a relation of the form
Re
L
=
ρ
vL
(
)
(
)
Nu
=
fx
,Re,Pr
or
Nu
=
f
Re ,Pr.
(1.4 0)
(1. 36)
x
x
L
L
µ
In most cases these relations are based on an empirical fit of the
equation to experimental data.
he
Grashof number
is defined by the dimensionless ratio
where all of the constitutive properties refer to the fluid: ρ is the
density (kg/m
3
),
v
is a representative flow velocity (m/s), and μ
is the viscosity (
N
·s/m
2
). An appropriate physical dimension of
the interface is indicated by
L
. The Reynolds number is a pri-
mary property applied to describe forced convection processes.
It describes the ratio of the inertial and viscous forces associ-
ated with the fluid low. For low
Re
values the low is dominated
by the viscous resistance resulting in a laminar boundary layer
in which the movement of fluid is highly ordered. High values
of
Re
have a much larger inertial component, which produces
a turbulent boundary layer. The magnitude of convective heat
transfer is strongly influenced by whether the boundary layer is
laminar or turbulent. The geometry of the interface and bound-
ary layer also plays an important role in the convection process.
Accordingly, the Reynolds number can be written in terms of
either an effective diameter,
D
, for an internal flow geometry, or
an interface length,
L
, for an external flow geometry. The transi-
tion between the laminar and turbulent regimes is defined in
terms of a threshold value for
Re
, and is very different for inter-
nal and external flow geometries. Thus, the
transition values
for
Re
are given as
(
)
3
=
β−
ν
gTTL
(1.41)
s
∞
Gr
L
2
where
g
is the acceleration of gravity (1/s
2
), and β is the volumet-
ric thermal expansion coefficient of the fluid (1/K). β is given by
the relationship
1
∂ρ
∂
β=−
ρ
(1.4 2)
T
p
which, for an ideal gas becomes
1
β=
T
(1.43)
where the temperature is given in absolute units (K). The Grashof
number is a primary property applied to describe free convec-
tion processes. It describes the ratio of the buoyant and viscous
forces associated with the fluid flow and is the equivalent of the
Reynolds number for free convection heat transfer. Accordingly,
for free convection processes the Nusselt number is calculated
from a relation of the form
trans
,int
=
ρυ
µ
D
Re
=
2300
(1. 37)
and
=
ρυ
L
5
Re
µ
=×
510.
(1. 38)
(
)
(
)
(1.4 4)
Nu
=
fxGr
,
,Pr
or
Nu
=
fGr
,Pr.
trans
,ext
x
x
L
L
There are many correlation equations of the type given in
Equations 1.40 and 1.44 for forced and free convection, respec-
tively. Comprehensive compendia of these relations are available
in dedicated topics (Bejan 2004; Kays, Crawford, and Weigand
2004; Incroprera et al. 2007). Only the most generally used rela-
tions are presented in this chapter, with a twofold purpose: to
illustrate the format of the correlations for various convective
domains and to provide a basic set of correlations that can be
applied to the solution of many frequently encountered convec-
tion problems.
he
Prandtl number
is defined by the dimensionless ratio
c
µ
=
ν
α
p
Pr
=
(1. 39)
k
where all of the constitutive properties refer to the fluid. The
symbol ν
is the kinematic viscosity (m
2
is which is the ratio
of the dynamic viscosity and the density. The Prandtl num-
ber describes the ratio of momentum diffusivity to thermal