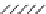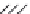Geoscience Reference
In-Depth Information
Fig. 10.19 Sketch illustrating the calculation of the drained volume as the area outside the
η
=
η
(
x
) curve, that is
the water table height at a given instant in time
t
. This can be done by integrating either the elemental
area (
xd
η
) or the elemental area (
D
− η
)
dx
. The point
x
=
0 is where the ground water exits the
aquifer, and
B
is the breadth of the aquifer or the distance of the divide from the stream channel.
WT
D
D
c
=0
x=0
x=B
Fig. 10.20 Sketch illustrating the short-time water table configuration in an unconfined hydraulic aquifer, while
the boundary condition at
x
=
B
can be assumed to have no effect. The boundary conditions for this
situation are Equations (10.53), and Boltzmann's transform is applicable.
(see Figure 10.20). If initially the aquifer is fully saturated, it can be assumed that as the
outflow starts the flow condition at
x
0 is not “felt” further away from the channel,
and that the flow proceeds as if the aquifer is infinitely wide. Subsequently, however, as
drainage continues, the effect of this condition diffuses, not unlike a wave, away from
x
=
B
, the short time solution gradually becomes invalid.
The boundary conditions, describing flow from such an infinitely wide, initially saturated
aquifer into an empty channel, can be formulated as
=
0, and as it approaches
x
=
η
=
0
x
=
0
t
≥
0
(10.53)
η
=
Dx
>
0
t
=
0
Similarity considerations
Like in the sorption problem in Section 9.2, the nature of the semi-infinite flow domain
and these boundary conditions imply a certain symmetry; because the aquifer must be
empty after a very long time of drainage, the water level for
t
→∞
, is the same as at
the water will
not “feel” the effect of the drainage and will remain at the original level it had at
t
x
=
0 for all times; similarly, very far from the channel, i.e. for
x
→∞
=
0.