Graphics Reference
In-Depth Information
k
k
l :
V
Æ
E
V
(C.15)
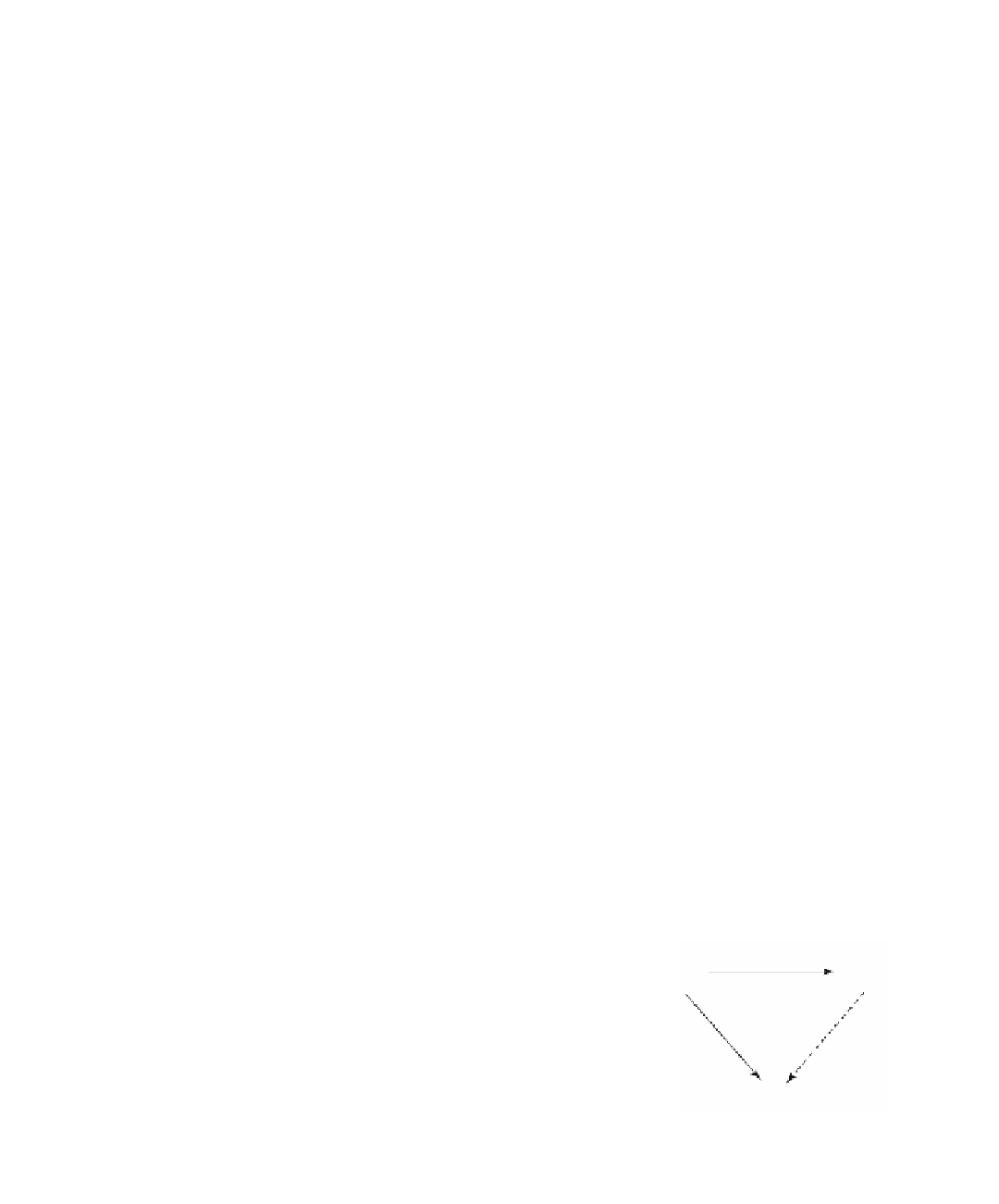
be the composite of the maps
æÆææÆ
Alt
k
k
k
V
T
V
æ
E
V
.
C.6.13. Theorem.
Let g :
V
k
Æ
W
be an alternating multilinear map. Then there is
a unique linear transformation h : E
k
V
Æ
W
so that g = h
l. In fact, the map
gh
Æ
defines an isomorphism between alternating multilinear maps on
V
k
and linear maps
on E
k
V
. As a special case we get an isomorphism
()
Æ
(
)
L
k
k
y :
V
E
V
*,
where
(
)
=
()
(
)
g
vv
,
,...,
v
y
g
v v
Ÿ
Ÿ◊◊◊Ÿ
v
(C.16)
12
k
1
2
k
for all g ΠL
k
(
V
) and
v
i
Œ
V
.
Proof.
See Figure C.5. The theorem is an easy consequence of Theorem C.6.9. For
a proof see [AusM63].
Theorem C.6.1 and C.6.2 remain true if we replace “multilinear map” with “alter-
nating multilinear map”.
Alternating multilinear maps admit a product very much like the alternating
tensor product.
Definition.
The
alternation map
k
()
Æ
k
()
Alt
:
L
V
L
V
is defined by
1
Â
()
=
(
)
Alt T
sign
ss
T
,
if k
≥
1
,
k
!
s
Œ
S
k
=
T fk
,
=
0
.
l
E
k
V
V
k
h
g
The universal property of the space E
k
V
.
Figure C.5.
W