Graphics Reference
In-Depth Information
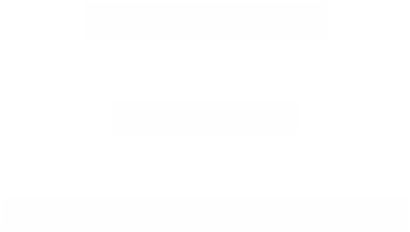
defined by the condition that
(
)
(
)
=
()()
ja
ƒ
b
uv
ƒ
a
uv
b
for all aŒ
U
*, bŒ
V
*,
u
Œ
U
, and
v
Œ
V
.
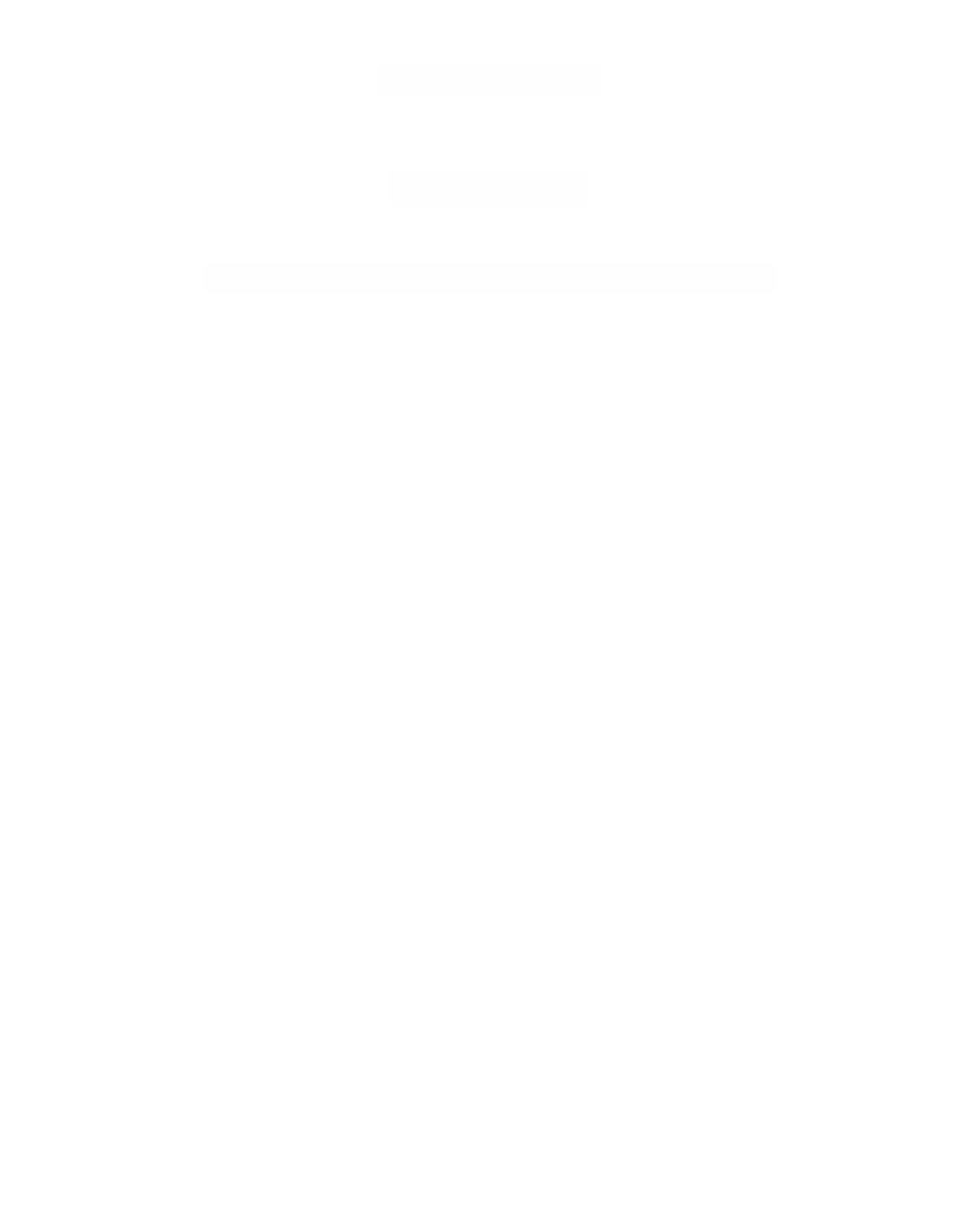
(2) There is a unique isomorphism
(
Æ
(
)
k
k
j :
T
V
*
T
V
*
(C.9a)
defined by
(
)
(
)
=
() ()
◊◊◊
()
ja
ƒ
a
ƒ◊◊◊ƒ
a
vv
ƒ
ƒ◊◊◊ƒ
v
a
v v
a
a
v
(C.9b)
1
2
k
1
2
k
1
1
2
2
k
k
for all a
i
Œ
V
* and
v
i
Œ
V
.
Proof.
To prove part (1), note that the universal factorization property of tensor
products implies that j is induced by the bilinear map
(
)
f:
UV UV
*
¥Æƒ
*
*
(C.10a)
defined by
(
)
(
)
=
()()
f ab
,
uv
ƒ
a
uv
b
.
(C.10b)
To show that j is an isomorphism, show that the vectors j(
u
i
* ƒ
v
j
*) are linearly inde-
pendent for some dual basis
u
i
*
for
U
* and
v
j
*
for
V
*. See [AusM63] or [KobN63]. Part
(2) is an easy generalization of part (1).
Because of Theorem C.6.5 we shall not distinguish between tensor products like
U
* ƒ
V
* and (
U
ƒ
V
)* whenever it is convenient. Note that another way to prove the
existence of an isomorphism between these spaces is to show that ((
U
ƒ
V
)*,f) is a
tensor product for
U
and
V
, where f is the map in (C.10b). The same comment applies
with regard to the existence of an isomorphism between T
k
(
V
*) and (T
k
V
)*. We did
not do this because for us it is convenient to be explicit about the formula (C.9b) for
the isomorphism j.
Using the isomorphism y and j in Theorems C.6.4 and C.6.5(2), respectively, gives
us an isomorphism
-
1
k
(
Æ
k
()
yj
:
T
V
*
L
V
.
(C.11)
C.6.6. Theorem.
The isomorphisms in (C.11) induce an isomorphism of algebras
(
Æ
()
F :
T
V
*
L
V
,
(C.12a)
where
Fa
(
ƒ
a
ƒ◊◊◊ƒ
a
)
=
a
ƒ
a
ƒ◊◊◊ƒ
a
(C.12b)
1
2
k
1
2
k
for all a
i
Œ
V
*.