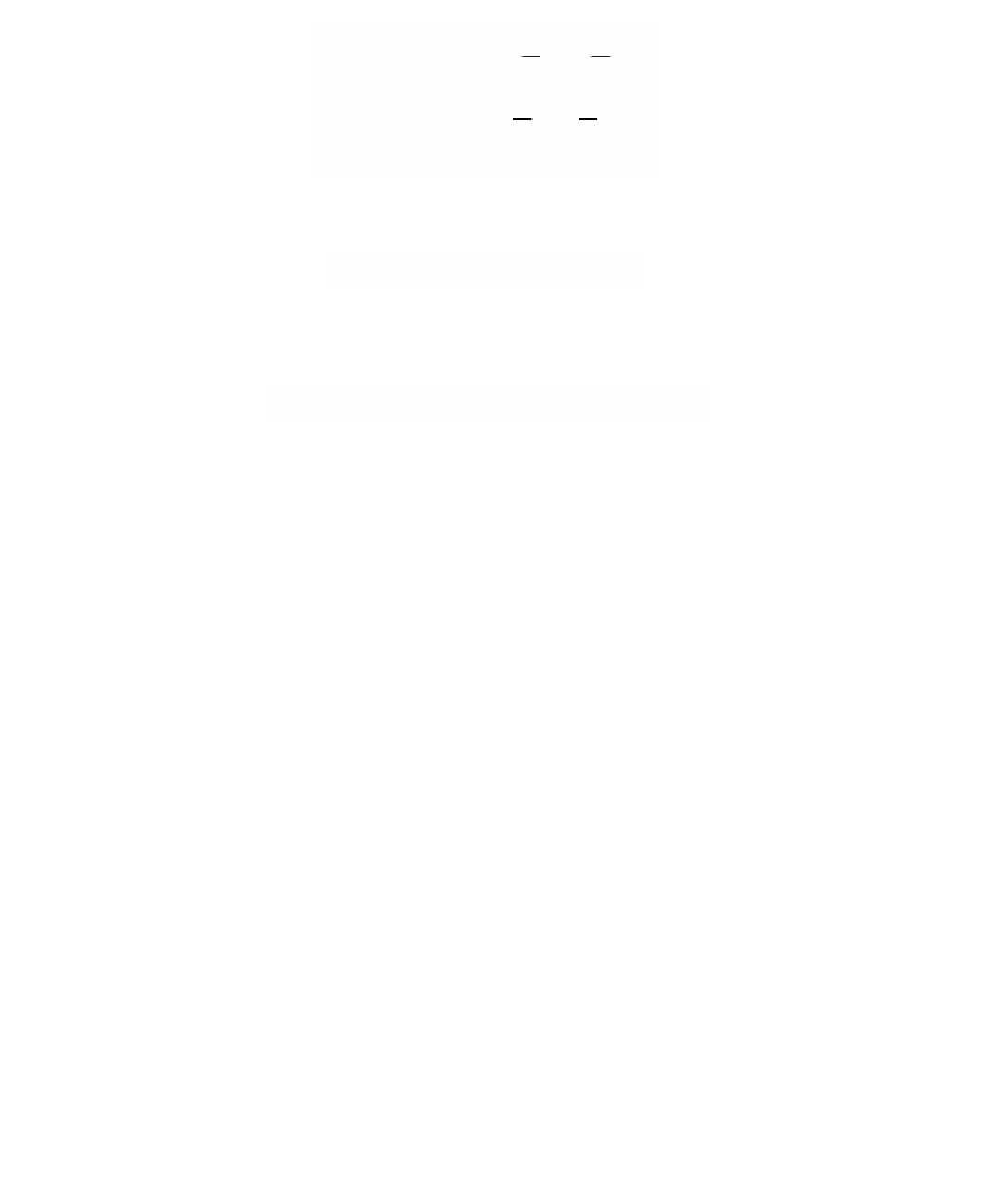
Graphics Reference
In-Depth Information
2
È
˘
YYY
X
Y
X
Y
Ê
Ë
ˆ
¯
2
2
2
X
-
3
+
2
=
-+
3
2
Í
˙
Î
˚
Y
X
XYX Y
X
Y
Ê
Ë
ˆ
¯
Ê
Ë
ˆ
¯
2
=
Y
-
1
-
2
(
)
(
)
.
=-
-
2
10.5.4. Proposition.
Let (a
1
,a
2
,...,a
n
) Πk
n
. Every polynomial f Πk[X
1
,X
2
,...,X
n
]
can be written in the form
(
)
(
)
fb X af
=+
111
...
-
++ -
X af
,
nnn
where b Πk, f
i
Πk[X
1
,X
2
,...,X
n
].
Proof.
The proposition follows from the fact that
[
]
=
[
]
kX X
,
,...,
X
kX
-
a X
,
-
a
,...
X
-
a
,
12
n
1 12 2
n
n
so that k[X
1
,X
2
,...,X
n
] can be thought of as a polynomial ring in X
1
- a
1
,...,
X
n
- a
n
.
10.5.5. Theorem.
If k is an algebraically closed field, then any nonconstant poly-
nomial f in k[X
1
,...,X
n
], n > 1, has an infinite number of zeros.
Proof.
We shall sketch a proof for the case n = 2 and leave the general case to the
reader. Since f is not constant, we may assume without loss of generality that f(X,Y)
is of the form
(
)
=
()
r
()
r
-
1
()
()
[]
...
+
a
,
a
Œ
k
,
fXY
,
a YX
+
a
YX
+
Y
Y
Y
r
r
-
1
0
i
where r > 0 and a
r
(Y) π 0. If the degree of a
r
(Y) is s, then a
r
(Y) has at most s roots.
Since k is infinite, there are infinitely many c in k so that a
r
(c) π 0. Consider
()
r
()
r
-
1
++
()
=
acX a
+
cX
...
ac
0
.
r
r
-
1
0
This equation has a root b because k is algebraically closed. By definition, f(b,c) = 0.
To finish the proof one simply needs to show that the infinite number of choices for
c lead to an infinite number of solutions to f(X,Y) = 0.
10.5.6. Theorem.
Let k be an arbitrary field, f an irreducible polynomial in
k[X
1
,...,X
n
], and g an arbitrary polynomial in k[X
1
,...,X
n
]. If f does not divide g,
then there are only a finite number of solutions to the equations
(
)
=
(
..,
X
)
=
fX
,...,
X
gX
,.
0
.
1
n
1
n
Proof.
Again, we shall only give a proof for the case n = 2 and leave the general case
to the reader. By hypothesis f cannot be constant and so we may assume without loss