Graphics Reference
In-Depth Information
[
]
2
2
g≤=
Lu
¢ +
2
Mu v
¢ ¢ +
Nv
¢
n
[
]
[
]
11
12
1
1
22
12
11
22
1
2
22
22
+≤+
u
G
u
¢
+
2
G
u v
¢
¢ +
GF
v
¢
+≤+
v
G
u
¢
+
2
G
u v
¢
¢ +
GF,
v
¢
u
v
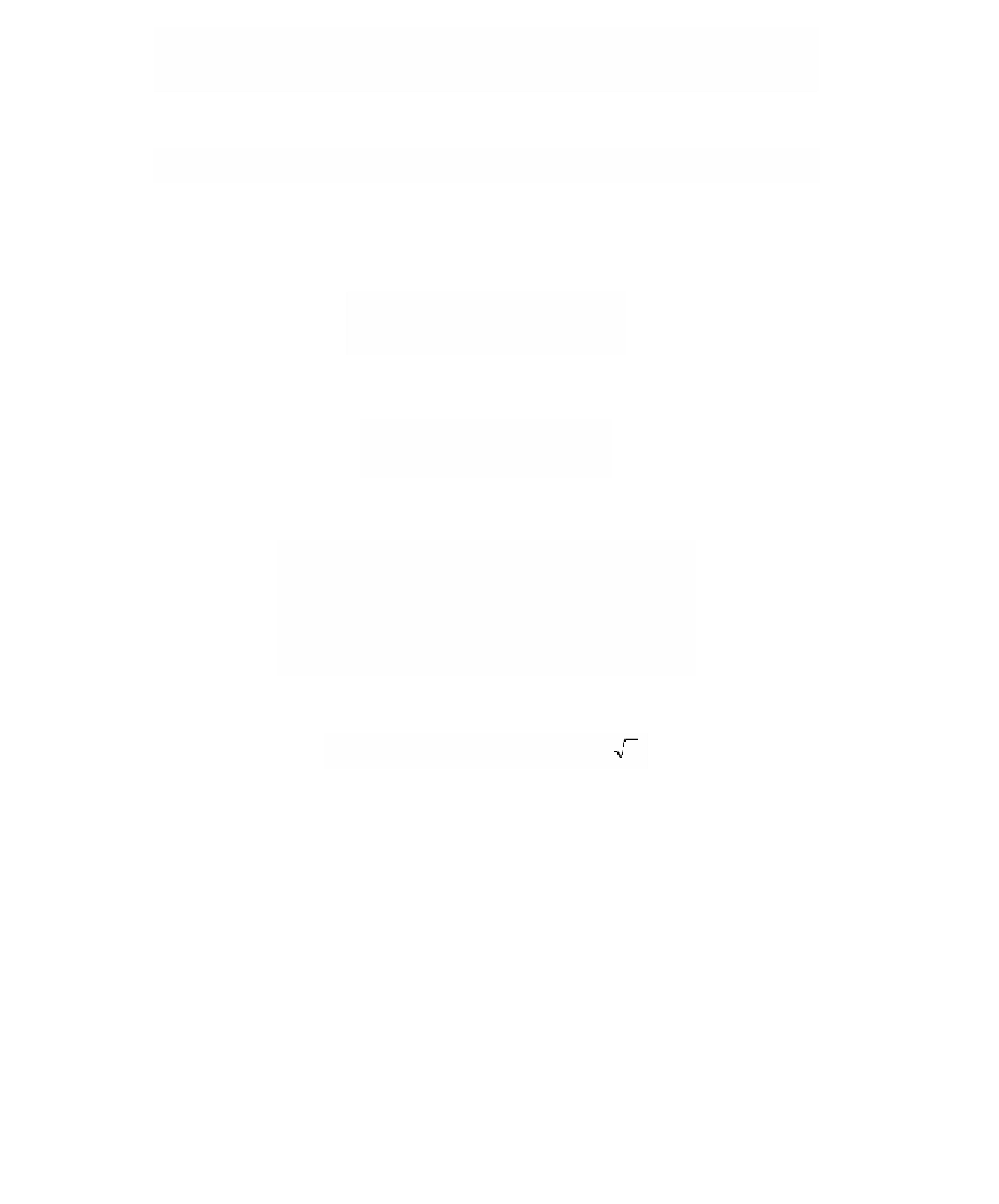
so that equation (9.65) clearly implies that
[
]
[
]
12
1
12
22
2
22
k
g
nu u
S
=≤+
G
¢
+
2
G
uv
¢
¢ +
G
v
¢
F
+≤+
v u
G
¢
+
2
G
uv
¢
¢ +
G
v
¢
F
.
u
v
11
12
22
11
12
22
To make the pattern more apparent, we change our notation slightly and rename our
parameters u and v to x
1
and x
2
, respectively, so that we can rewrite the last equation
using summation notation as
2
È
Í
2
˘
˙
Â
Â
k
k
g
n
S
=
x
¢¢ +
G
x x
¢
¢
F
.
(9.71)
k
ij
k
ij
k
=
1
ij
,
=
1
(F
1
and F
2
are the partials F
u
and F
v
, respectively.) But, using Equation (9.69),
2
Â
n
=¥¢ =
n
g
x
m
¢
n
¥
F
.
S
m
m
=
1
Therefore, if we let c
km
=
n
•(F
k
¥F
m
), then
2
2
Ê
Á
È
˘
ˆ
˜
∑
Â
Â
=
(
)
∑=
i
k
kk
g
nn
x
¢¢ +
G
xx
¢
¢
F
n
Í
˙
g
S
S
k
i
j
k
S
Î
˚
k
=
1
ij
,
=
1
2
2
Ê
Á
È
Í
˘
˙
ˆ
˜
Â
Â
k
=
x
¢¢ +
G
x x
¢
¢
x c
¢
.
k
ij
kkm
ij
km
,
=
1
ij
,
=
1
Finally, since
c
==
c
0
and
c
=-=,
c
g
11
22
12
21
we have shown that k
g
depends only on the Christoffel symbols, which are metric
invariants by equation (9.59), and g. The theorem is proved.
Moving on to property (2) of straight lines, we can see from Example 9.10.8 that
a geodesic in
S
2
, as we have defined one, can start at a point, go round and round a
great circle, and finally end up at that point again, so that it is
not
necessarily the
shortest curve between any two points. On the other hand, they do satisfy property
(2) locally. One can prove the following:
Let
S
be a surface in
R
3
. Let h : [a,b] Æ
S
be a regular curve.
9.10.11. Theorem.
(1) If h(t) is a geodesic, then there is an e>0 with the property that if |s
1
- s
2
|
<ethen g |[s
1
,s
2
] is a curve of shortest length from g(s
1
) to g(s
2
).
(2) If h(t) is a curve of shortest length from h(a) to h(b), then h(t) is a geodesic.