Graphics Reference
In-Depth Information
gluing the ends together like we do for the torus, we bend one end and bring it to the
inside of the tube (Figure 6.24(b)), and finally glue that end to the other one from the
inside (Figure 6.24(c)). Of course, as can be seen from Figure 6.24, this involves pierc-
ing the tube. As a result we do not get a legitimate surface since we have a self-inter-
section along a circle (marked
X
in Figures 6.24(b) and (c)). Unfortunately, we cannot
eliminate this singularity because the surface
K
that we were trying to define, like the
projective plane, cannot be imbedded in
R
3
. We are seeing
K
after it has been “pushed”
into
R
3
. On the other hand, if we had a fourth dimension, then we could grab one
part of the surface near the intersection
X
and lift it up into the fourth dimension,
thereby removing any intersection and creating a real surface without singularities.
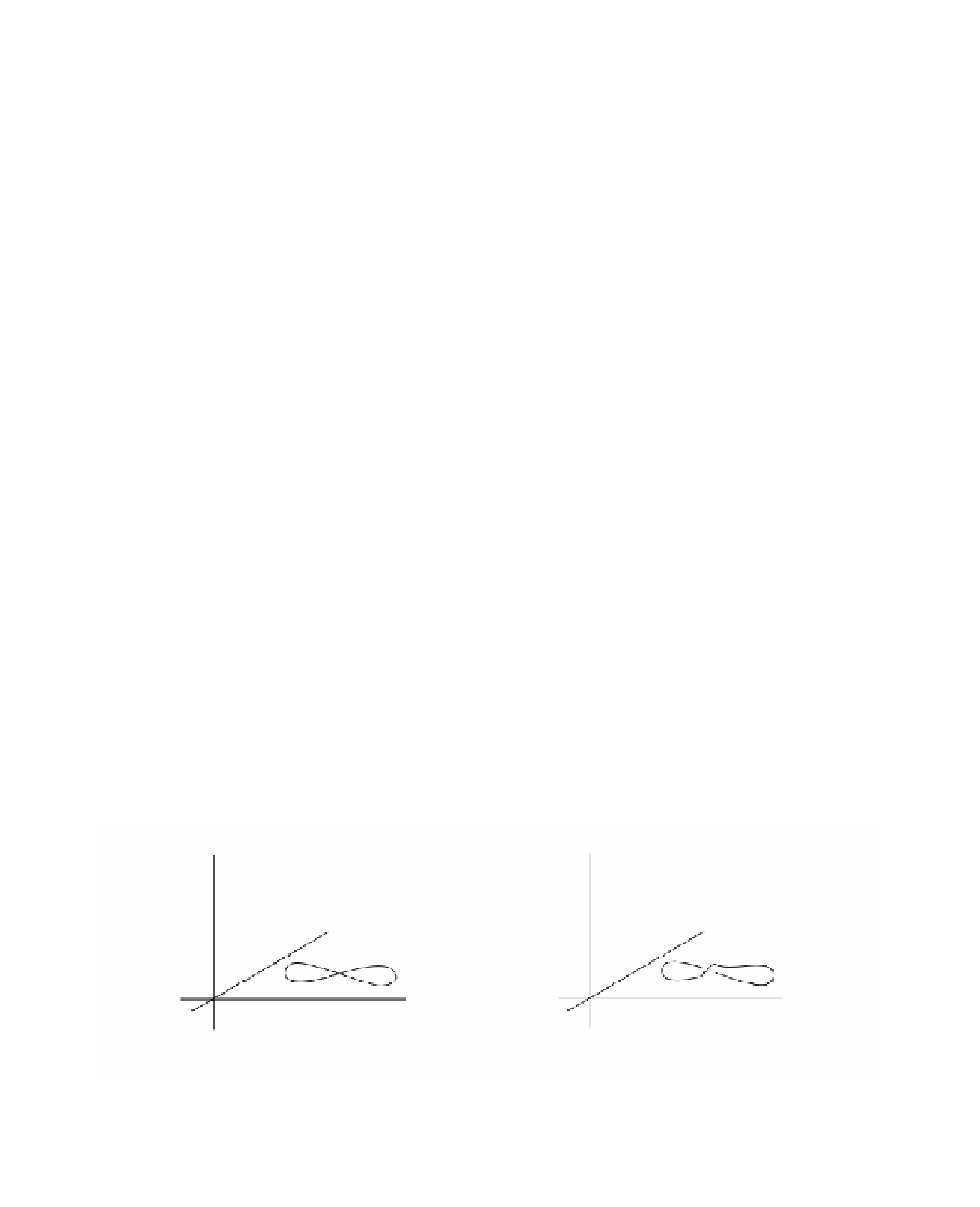
This is in analogy with the way that one can remove the self-intersection of a circle
immersed in the plane as a figure eight. We can remove the self-intersection by lifting
one part of the circle near the intersection up into the third dimension. See Figure
6.25. If one can understand how a two-dimensional person could try to visualize that
three-dimensional construction, then one should be able visualize what the surface
K
looks like. It is clear from the construction, that
K
can also be described as a square
with sides identified as shown in Figure 6.24(d). This means that we can give the fol-
lowing precise definition of a Klein bottle:
Any surface with symbol A
1
A
2
A
1
A
-1
is called a
Klein bottle
.
Definition.
Proposition 6.5.11.
The Klein bottle
K
is a nonorientable surface that is homeo-
morphic to
P
2
#
P
2
.
Proof.
Represent the Klein bottle via the labeled polygon shown in Figure 6.26. If
we cut
K
along the lines indicated by
c
and
d
, then we get two Moebius strips with
the shaded region being one of them. This shows that
K
is gotten by taking two
Moebius strips and gluing them together along their boundaries. This is precisely what
we have in the case of
P
2
#
P
2
, since the projective plane can be gotten by gluing a
disk to a Moebius strip along their boundaries.
Intuitively, we can see that the Klein bottle is nonorientable because it is one-
sided. Here we are thinking of the surface as made of, say, paper and we are walking
along it. See our discussion of the Moebius strip in Section 1.6. Because of the one-
z
z
y
y
x
x
(a)
(b)
Figure 6.25.
Eliminating a self-intersection using a third dimension.