Graphics Reference
In-Depth Information
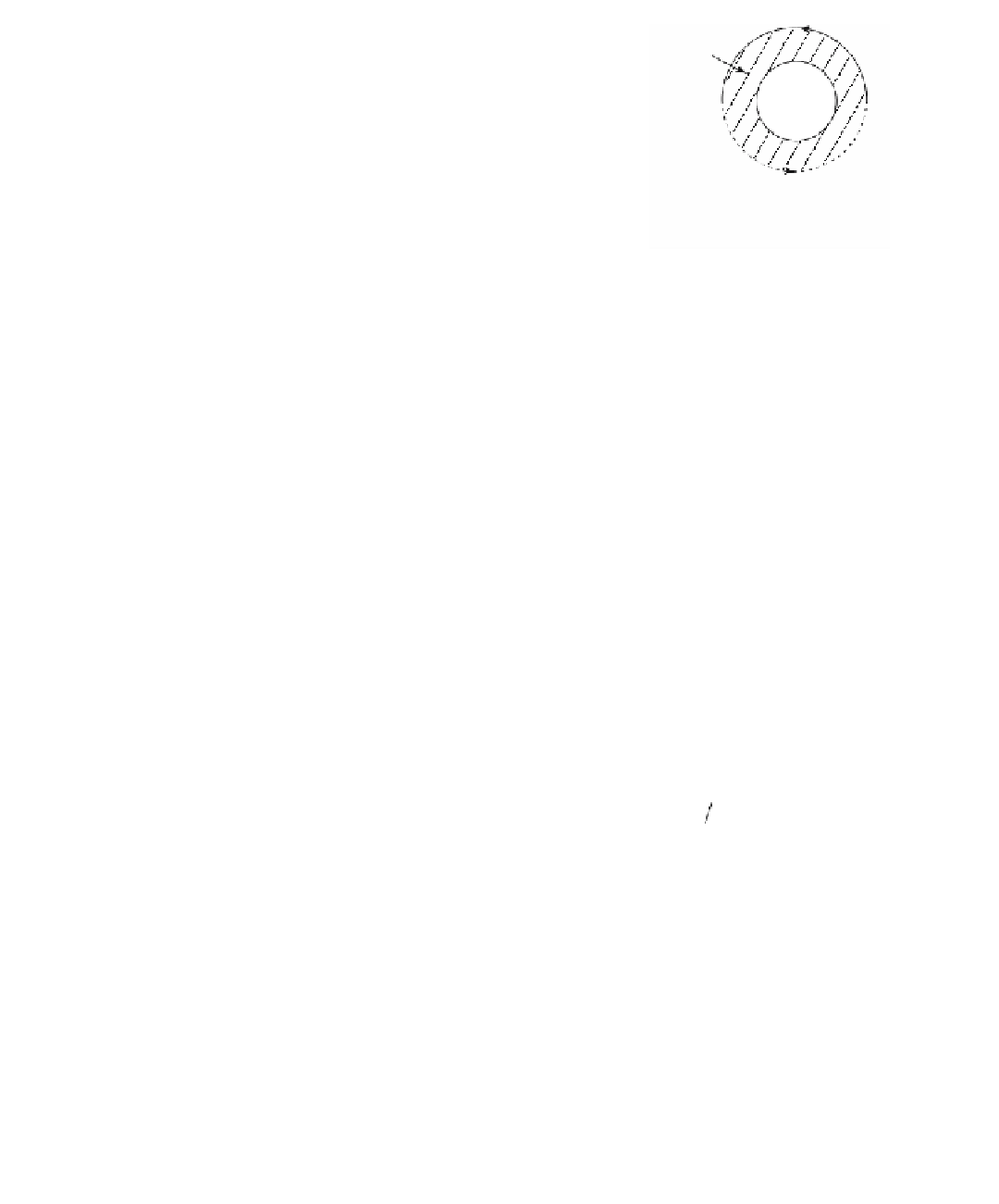
Figure 5.15.
Another way to visualize the projective plane.
Moebius
strip
a
disk
a
Disk glued to Moebius strip
along their boundary
that
P
n
can be thought of as
S
+
/~, where ~ is a restricted version of the equivalence
relation in the third definition above, namely,
p
~-
p
for
p
S
n-1
. Our characteriza-
tion now follows from the observation that the only identifications that are taking
place are on the boundary of
S
+
and that the interior of
S
+
projects in a one-to-one
fashion onto the interior of the disk
D
n
.
In the case of the projective plane, there is another well-known identification.
A fifth definition of P
2
: The projective plane is the union of the Moebius strip and
a disk where we identify their boundaries, which are just circles.
Justification:
Consider the shaded region in Figure 5.15, which is a “collar” of the
boundary of the unit disk. A little thought should convince the reader that under
the identification described in the fourth definition, this shaded region is just the
Moebius strip.
We now have four different ways of looking at the topological space
P
n
(five,
in the case of the projective plane). In each case we used a quotient topology of
Euclidean space. Alternatively, one can define this topology by defining a metric on
P
n
.
Let
p
= [
x
] and
q
= [
y
] be points of
P
n
, where
x
,
y
R
n+1
. Define the
Definition.
distance between
p
and
q
, denoted dist (
p
,
q
), by
xy
xy
∑
(
)
=
dist
pq
,
d
,
where
cos
d
=
and
0
££
d
p
2
.
It is easy to see that dist(
p
,
q
) is well defined and does not depend on the repre-
sentatives
x
and
y
that are chosen for
p
and
q
, respectively. It is just the angle between
the two “lines”
p
and
q
. The function dist(
p
,
q
) is in fact a metric on
P
n
and makes
P
n
into a metric space.
Let
L
be the line in
R
2
5.9.1. Example.
defined by -x + 2y + 1 = 0. To find the
points of
P
2
that are “near” the ideal point
L
associated to the family of lines
parallel to
L
.
Solution.
Let
p
= [X,Y,Z] be any point of
P
2
. Since
L
= [-2,-1,0], a simple com-
putation using the definition of distance shows that the distance d between the two
points satisfies