Graphics Reference
In-Depth Information
atives of order r exist
and
are continuous for 1 £ R £ k. The set of such functions is
denoted by C
k
(
A
). If f :
A
Æ
R
m
, then f is said to be of
class C
k
if all the component
functions are of class C
k
. C
1
functions are often called
continuously differentiable
functions. If f is of class C
k
for all k, then we say that f is of
class C
•
. A C
•
function
is also called a
smooth
function.
4.3.10. Theorem.
Let
U
be an open subset of
R
n
. If f :
U
Æ
R
m
is differentiable at
p
,
then the jth partial derivatives D
j
f
i
(
p
) exist for all i and the m ¥ n matrix (D
j
f
i
(
p
)) is
the
transpose
of what we have called the matrix for the linear transformation Df(
p
)
with respect to the standard bases of
R
n
and
R
m
.
Proof.
This is an easy consequence of the definitions and the chain rule. See [Spiv65]
for details.
Definition.
With the notation and hypotheses of Theorem 4.3.10, the matrix (D
j
f
i
(
p
))
is called the
Jacobian matrix
and will be denoted by f ¢(
p
). Using the more common
partial derivative notation,
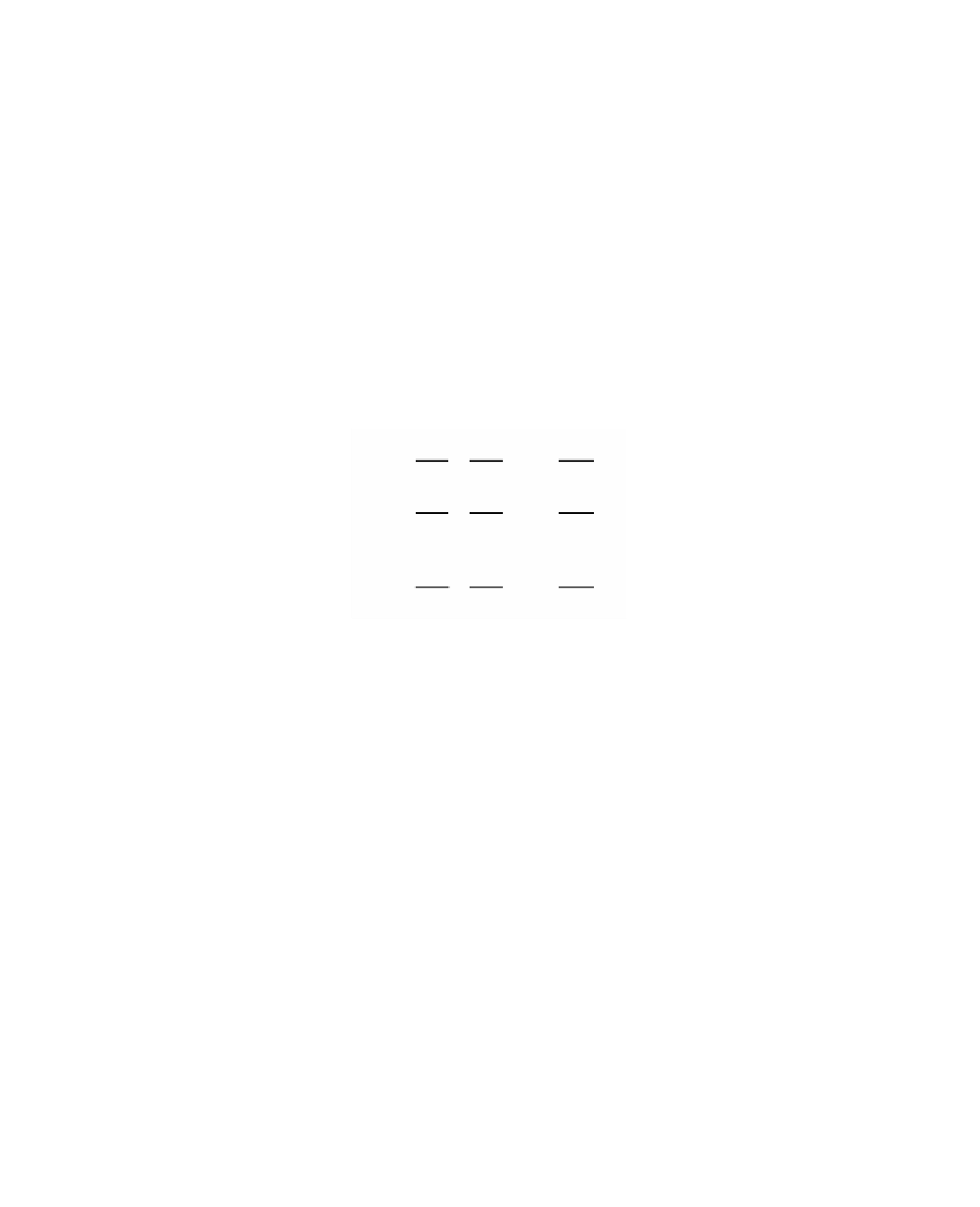
∂
∂
f
x
∂
∂
f
x
∂
∂
f
x
Ê
ˆ
1
1
1
2
1
K
Á
Á
Á
Á
Á
Á
Á
˜
˜
˜
˜
˜
˜
˜
n
∂
∂
f
x
∂
∂
f
x
∂
∂
f
x
2
1
2
2
2
K
f
¢=
.
n
M
M OM
K
∂
∂
f
x
∂
∂
f
x
∂
∂
f
x
mm
m
n
Ë
¯
1
2
If m = n, then the determinant of the n ¥ n Jacobian matrix f ¢(
p
) is called the
Jaco-
bian
of f at
p
. If m = 1, then it is often convenient to treat the 1 ¥ n matrix f ¢(
p
) as a
vector in
R
n
.
Note.
With our definition, the matrix f ¢(
p
), thought of as a transformation, will act
on vectors on the
right
and not on the left as is the case everywhere else in this topic.
The reason for departing from our usual convention regarding the matrix of a linear
transformation and using the transpose here is to be compatible with how most people
define the Jacobian matrix in terms of partials. If m = n = 1, the new notation f ¢(
p
)
will also be compatible with the old notation for the derivative of a function of one
variable if we think of a number as a 1 ¥ 1 matrix.
4.3.11. Theorem.
Let
U
be an open subset of
R
n
and let f :
U
Æ
R
m
and assume that
each D
j
f
i
exists in a neighborhood of a point
p
and is continuous at
p
. Then f is
differentiable at
p
.
Proof.
See [Spiv65].
Assuming that one can take partial derivatives of ordinary real-valued functions,
we now show via some examples how easy it is to find derivatives of vector-valued
functions and how the derivatives really do correspond to approximating the func-