Cryptography Reference
In-Depth Information
3.8 Torsion Points
Every p
o
int on an elliptic curve
E
has a finite order or an infinite order. Let
PE
Î
where
K
is the algebraic closure of
K
, and let
n
be a small integer; then there exists a
finite subgroup such that
( ,
(3.35)
to
En
[] {
=Î
P EKnP
( ) |
=Í
}
En
[]
Î
Hence,
n
is the order of
P
. On the contrary, if no such
n
exists for a
PEK
()
(we will
never get
by adding
P
to itself ), then the order of the point is infinite.
2
3
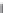
Example 3.1.
Let us consider the elliptic curve
EK y
/:
=+
where
K
=
F
11
x
3,
x
(Figure 3.9).
The set of rational points on
E
is
{[0,0],[1,9],[1,2],[2,5],[2,6],[3,5],[3,6],[6,5],[6,6],
[7,1],[7,10], }
Therefore, #
E
(
K
)
=
12. Referring to Eq. (3.20), the value of
t
=
0.
Hence the elliptic curve
2
3
=+
defined over
F
11
is supersingular. Table
3.4 shows the order of the torsion points in
EK y
/:
x
3
x
2
3
EK y
/:
=+
whe
re
K
=
F
11
.
x
3
x
Let
Ey
:²
=++
be an elliptic curve and let
x
³
Ax B
Î
be the roots of
(, , )
rr r
123
the cubic equation
E
. Then
2
y
=- - -
(
x
r
)(
x
r
)(
x
r
)
(3.36)
1
2
3
If a point
P
reaches infinity at 2
P
, then a line through the
P
is a tangent (Figure
3.5). Hence, we get the subgroup with second-order torsion points.
(3.37)
E
[2]
=
{( ,0),(
r
r
,0),(
r
,0),
}
tor
1
2
3
12
y
10
8
6
4
2
0
0
1
2
3
4
5
6
7
8
9
10
11
12
x
EKy x x
where
K
=
F
11
/:
2
3
3,
Figure 3.9.
=+





















































































































Search WWH ::

Custom Search