Geology Reference
In-Depth Information
The delta function is often used in (recipe-oriented) “operational” or
“transform calculus.” For us, it suffices to list its two best known properties,
(x-x
s
) dx = 1
(1.100)
and
P(x) (x-x
s
) dx = P(x
s
)
(1.101)
where the ... dx is carried out over any domain containing x
s
and P(x) may be
any function. Topics dealing with “distributions” are available, but we need not
delve further, e.g., Lighthill (1959). If more than one force appears, multiple
delta functions simply appear on the right side. It is clear from Figure 1.4 that F
is responsible for an abrupt change in the slope u/ x. In particular, while the
displacement u itself is “continuous” or “single-valued” through the point of
contact (and hence, the speed and acceleration), the slope u/ x takes on two
different values at the left and right: it is “double-valued.”
That is, a point force (or delta function) produces a “slope discontinuity” or
a “jump in the first spatial derivative” of the dependent variable u. This jump is
conventionally denoted by enclosing the discontinuous quantity in rectangular
brackets; in this example, we have [ u/ x] . The exact magnitude can be
obtained from momentum considerations, but we instead obtain it by integrating
Equation 1.99 from x = x
s
- to x = x
s
+ where is a small positive number,
2
u/ t
2
dx +
u/ t dx
l
- T
2
u/ x
2
dx +
l
g dx = F(t)
(x-x
s
) dx
(1.102)
Integration limits are omitted for brevity. It is clear that, if our string does not
break, the acceleration
2
u/ t
2
takes on the same value at either side of the force;
thus, its integral vanishes identically as tends to zero (the same vanishing
applies to the u/ t velocity and gravity terms, and the elastic force had we
included it in our formulation). Now, the Dirac delta function is always defined
with the property that its areal sum is
exactly
unity (see Equation 1.100). Since
the slope
u/ x can and will change through x = x
s
, as shown in Figure 1.4, we
conclude that Equation 1.102 reduces to
[ u/ x]
x=xs
u/ x |
x=xs+
- u/ x |
x=xs-
= - F(t)/T
(1.103)
Thus, an applied force F(t) will produce a jump in slope in the amount -F(t)/T
that is independent of
l
. Suppose a hypothetical mechanical device creates
internal slope discontinuities [ u/ x] of magnitude -F(t)/T at x = x
s
. Then this
device is modeled by Equation 1.99. Since Figure 1.4 shows a single force, or a
single delta function, we refer to the excitation as a “monopole.”
















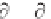
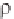


















Search WWH ::

Custom Search