Geology Reference
In-Depth Information
transient state and propagate outward. Finite boundaries interrupt this
propagation, reflecting part of the energy back toward the source; repeated
indefinitely, standing waves appear after sufficient time.
1.4.3 Combined standing and propagating waves.
A wave system can be standing
and
propagating. Consider a semi-infinite
string (0 < x < ) attached to a constraint at x = 0. If a point excitation at x = x*
> 0 oscillates sinusoidally at constant frequency, then 0 < x < x* contains a
standing wave, while x > x* hosts the propagating wave. This might model
waves from a MWD pulser in a drillstring where the pulser is a finite distance
from the drillbit.
1.4.4 Characterizing propagating waves.
We have considered two simple wave models so far, the undamped and
damped wave equations in “canonical form.” Here we will characterize
propagating waves in greater detail. Thus, we will consider the undamped
transverse vibrations of a string satisfying
l
2
u/ t
2
- T
2
u/ x
2
= 0 and
dissipative waves satisfying
l
2
u/ t
2
+ u/ t - T
2
u/ x
2
= 0 (note that c
2
=
T/
l
). Here,
l
, , and T denote lineal mass density, damping factor and tension
(a formal derivation is given later). In going from the undamped to the damped
model, we uncovered new effects such as shape distortion and changes to wave
speed, but many more effects await discovery.
In order to understand wave propagation more fully, let us consider the
undamped model
l
2
u/ t
2
- T
2
u/ x
2
= 0, and assume a uniform, plane,
“monochromatic” wave solution u(x,t) = A sin (kx - t), where A is the “wave
amplitude,” k is the “wavenumber,” and is the “frequency.” The wavenumber
is the number of “wave crests” in a length 2 , whereas the frequency is the
number of oscillations in a time 2 . Substitution of u(x,t) = A sin k {x - ( /k) t}
in the wave equation yields
l
2
= Tk
2
. Thus, the solution for the speed /k =
(T/
l
) shows that both right and left-going waves are correctly found in the
substitution; a sinusoidal wave component stays sinusoidal as it propagates.
On the other hand, suppose we consider the more complicated situation in
which the string rests on an elastic base, so that our vibrations now satisfy the
equation
l
2
u/ t
2
+ u - T
2
u/ x
2
= 0. Here, the Greek letter (kappa)
describes the elastic constant of the foundation. Now, the substitution u(x,t) =
sin (kx - t) leads to
2
= ( T/
l
)k
2
+ /
l
, so that the speed /k = {(T/
l
)k
2
+ /
l
})/k depends on k. Thus, we find that waves having different lengths
propagate with different speeds. Such waves are said to be “dispersive,” as
opposed to being “nondispersive.” The equations
l
2
= Tk
2
and
2
= ( T/
l
)k
2
+ /
l
are “dispersion relations” because they describe wave dispersion.
Because a general disturbance consists of wave components with different






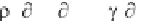






































Search WWH ::

Custom Search