Geology Reference
In-Depth Information
for the transient part u
d
(x,t) of the complete displacement field u(x,t)
If
the
surface can be represented by a stress-free end with u
d
(L,t)/ x = 0, while the
drillbit is forced to oscillate with the usual imposed displacement u
d
(0,t) = u
0
e
i t
, where the amplitude u
0
is real, simplifications are possible. The motivation
for complex exponentials was given in Chapter 1. Again, the notation u
0
e
i t
means that only the real part u
0
cos t (that is,
Re a l
{u
0
e
i t
}) of the complete
expression is significant. This device, owing to linearity, allows us to express
constraints in a simple manner.
We next consistently formulate the displacement problem in terms of an
amplitude function U(x) satisfying u
d
(x,t) = e
i
t
U(x). Equation 4.2.110 reduces
to an ordinary differential equation, namely,
U"(x) + {(
2
- i
)
}U(x) = 0
(4.2.111)
whose general solution takes the form
U(x) = A sin { (
2
-i )/E} x + B cos { (
2
-i )/E} x (4.2.112)
where A and B are constants of integration. At the bit, we therefore find that
u
d
(0,t) = e
i t
U(0) = e
i t
B = u
0
e
i t
, so that B = u
0
. Then the fact that U'(L) =
A{ (
2
-i )/E}cos { (
2
-i )/E}L - B{ (
2
-i )/E}sin { (
2
-i
)/E}L = 0 implies that the remaining constant satisfies A = B tan { (
2
-i
)/E}L = u
0
tan { (
2
-i )/E}L. The solution for U(x) is completely
determined. From Equation 4.2.112, it is clear that U(x) must be complex, that
is, U(x) = U
r
(x) + i U
i
(x) where U
r
(x) and U
i
(x) are real. We had started with
the convention that
Re a l
{u
d
(0,t)} =
Re a l
{u
0
e
i t
} = u
0
cos t.
Correspondingly, we have the solution
Re a l
{e
i t
U(x)} =
Re a l
{U
r
cos t - U
i
sin
t + i U
i
cos t + iU
r
sin t} = U
r
cos t - U
i
sin t where, again, the (complex)
function U(x) is obtained by simplifying Equation 4.2.112. It is apparent that
the formulation simplifications afforded by timewise periodicity do
not
justify
the obvious analytical complexities; more difficult boundary conditions, for
example, u
x
+ u
t
+ u = 0, are not easily handled. A fully transient numerical
formulation, as suggested here, will yield dynamically steady solutions if and
when they exist, but it will predict bit bounce and shock loadings as they arise.
The difference model deals with a real dependent variable whether or not
damping exists, and is simpler in this regard. Robust numerical approaches,
therefore, offer simplicity and complete generality for both linear and nonlinear
vibrations. Next, consider
nonuniform
drillstrings. We cite the work of Dareing
and Livesay (1968), who consider vertical drillstrings consisting of two sections.
The first section, marked by “1” subscripts, represents the drill collar occupying
0 < x < L
1
, where L
1
is the collar length. The second section, denoted by “2”














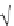



























Search WWH ::

Custom Search