Geology Reference
In-Depth Information
4.2.6.5 Other possibilities.
Other models are often used in pipe acoustics. For example, u
tt
+ u
x
= 0
describes the force balance due to an end stress with a mass. On the other hand,
u
x
+ u = 0 describes the interaction between an end stress and a spring, while
u
x
+ u
t
= 0 describes that between a damper and a stress. Later, we will study
the model u
x
+ u
t
+ u = 0 (see Equation 4.2.22); first, we consider the third
model discussed in this paragraph.
4.2.6.6 Simple derivative model model -
u
x
= u
t
at x = 0
.
With the function f(ct+x) given, the mathematical solution now takes the
form
u(x,t) = f(ct+x) + {( -c)/( +c)} f(ct-x) (4.2.43)
(see Morse and Feshbach (1953)). Since, u
x
= u
t
, it is clear that the product
u
x
u
t
= u
x
2
is nonzero, so that nontrivial energy transfer
does
occur at the bit
and formation interface. However, the solution shows that
u( 0 ,t) = { 1 + ( -c)/( +c)} f(ct) (4.2.44)
which vanishes on the average for a sinusoidal drillbit excitation. Thus, on the
average, the rate-of-penetration is unfortunately zero.
4.2.6.7 The general impedance mode -
u
x
+ u
t
+ u = 0.
This boundary condition, stated in Equation 4.2.22,
will
model nonzero
rate-of-penetration and bit-bounce. It contains the u
spring
and u
t
damper
elements suggested by Clayer, Vandiver, and Lee (1990), first postulated in
Chin (1988a,b) as part of a more general model. The effects of static and
transient WOB appear in the term u
x
. The presence of odd
and
even order
derivatives produces nonzero DC displacement levels when the incident
excitation is sinusoidal. This is crucial to modeling nonzero rate-of-penetration.
Consider now the linear impedance boundary condition
u
x
+ u
t
+ u = 0
(4.2.45)
at x = 0, a subset of the nonlinear statement in Equation 4.2.21.
Again with f(ct+x) given, we seek the function g(ct-x) such that u(x,t) =
f(ct+x) + g(ct-x) satisfies Equation 4.2.45 at x = 0. To this end, we substitute
u(x,t) = f(ct+x) + g(ct-x), u
x
(x,t) = f '(ct+x) - g'(ct-x), and u
t
(x,t) = cf '(ct+x) +
cg'(ct-x) into Equation 4.2.45, and evaluate the resulting expression at x = 0.
This leads to the ordinary differential equation ( c+ )f ' + f + ( c- )g' + g = 0
for g(ct). If F(s) and G(s) denote Laplace transforms of f and g with respect to
= ct, we obtain
G( s ) = - { (
c+ )s + } F(s) /{( c-
)s+ }
(4.2.46)







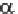


























Search WWH ::

Custom Search