Gravity field of the earth - Physical Geodesy - page 54

P

r

l

Ã

O

r'

dM

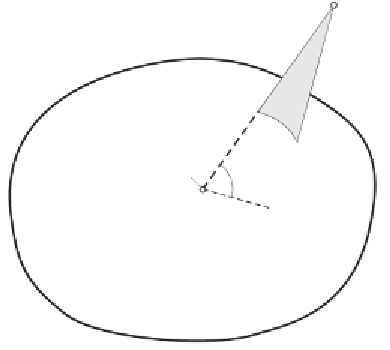
Fig. 2.9. Expansion into spherical harmonics

We now evaluate the coecients of this series. The gravitational potential
V is given by the basic equation (1-12):
V = G

dM
l

, (2-64)

earth

where we now denote the mass element by dM ; the integral is extended over
the entire earth. Into this integral we substitute the expression (1-104):

= ∞

r n
r n +1 P n (cos ψ ) ,

1
l

(2-65)

n =0

where the P n are the conventional Legendre polynomials, r is the radius
vector of the fixed point P at which V is to be determined, r is the radius
vector of the variable mass element dM ,and ψ is the angle between r and
r (Fig. 2.9).
Since r is a constant with respect to the integration over the earth, it
can be taken out of the integral. Thus, we get
V = ∞

r n +1 G
earth

1

r n P n (cos ψ ) dM . (2-66)

n =0

Writing this in the usual form as a series of solid spherical harmonics,
V = ∞

Y n ( ϑ, λ )
r n +1

, (2-67)

n =0