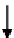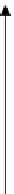Biology Reference
In-Depth Information
A
B
C
FIGURE 2-20.
Stable and unstable equilibria. Panel A depicts an unstable equilibrium, since the trajectories along
the vertical axis move away from the equilibrium. Panel B represents a repeller, because all
trajectories close to the equilibrium are forced away from it. Panel C depicts an asymptotically stable
equilibrium, attracting all trajectories.
An analytic condition, similar to the criterion for asymptotic stability, is
available to determine whether an equilibrium point is a repeller. As
before, we use the Jacobian matrix:
0
1
@
f
@
f
x
ð
x
0
;
y
0
Þ
y
ð
x
0
;
y
0
Þ
@
A
:
@
@
J
¼
@
g
@
g
x
ð
x
0
;
y
0
Þ
y
ð
x
0
;
y
0
Þ
@
@
The criterion, the first part of which we presented earlier, is
as follows:
Theorem: If det( J)
>
0, and trace( J)
<
0, then (x
0
, y
0
) is asymptotically stable.
If det( J)
>
0 and trace( J)
>
0, then (x
0
, y
0
) is a repeller.
The next predator-prey model we examine will exhibit cyclic or periodic
behavior. Although there will be equilibrium states, the trajectories do
not converge toward them. There is one more idea we need before we
can proceed.
Definition. A set U in the (x,y) plane is called a basin of attraction for the
system
dx
dy
dt
¼
dt
¼
f
ð
x
;
y
Þ;
g
ð
x
;
y
Þ
, if whenever a trajectory is in U at time
t
0
, it remains in U for all t
>
t
0
. In other words, if a trajectory ever enters
U, it stays there forever.
The main mathematical tool for our next example is:
Theorem (Poincar´-Bendixson). Suppose
dx
dy
dt
¼
dt
¼
f
ð
x
;
y
Þ;
g
ð
x
;
y
Þ
, has
U as a basin of attraction. Suppose that there is exactly one equilibrium point in
U and that point is repelling. Then the system has a periodic solution that
remains in U.
3
3. We call a solution (x(t),y(t)) periodic if is not an equilibrium state and there
exists T
>
0 such that
ð
x
ð
t
þ
T
Þ;
y
ð
t
þ
T
ÞÞ¼ðð
x
ð
t
Þ;
y
ð
t
ÞÞ
for all t
: