Geology Reference
In-Depth Information
(a)
(b)
T
/°C
P
H
2
O
=
2 × 10
8
Pa
1000
Melt
Melt + Na-rich
feldspar
ss
800
Homogeneous
feldspar
ss
0
f
h
1
2
P
H
2
O
/10
8
Pa
f
3
f
1
f
2
600
% NaAlSi
3
O
8
K-rich feldspar
ss
+
Na-rich feldspar
ss
0
20
Percentage of NaAlSi
3
O
8
by mass
40
60
80
100
KAlSi
3
O
8
(Or)
NaAlSi
3
O
8
(Ab)
Figure 2.6
Melting and subsolidus phase relations in the alkali feldspars (the system KAlSi
3
O
8
-NaAlSi
3
O
8
). The subscript 'ss'
denotes solid solution. (a) Perspective sketch of
PTX
HO
2
−− space, showing the isobaric section at 2 × 10
8
Pa illustrated in (b).
(b) Alkali feldspar phase relations at
P
HO
2
=× Pa. Horizontal ruling represents two-phase fields.
210
8
The diagram shows the liquidus and solidus of the
alkali feldspar series, which differ from those in
Figure 2.5 only in that they fall to a minimum melting
point in the middle of the series, rather than at one
end. As a result we get
two
leaf-shaped fields instead
of one. But interest is mainly in what happens in the
subsolidus region
. The 'homogeneous feldspar
ss
' field
immediately below the solidus means that here the
end-members are completely miscible in the solid
state: they form a complete solid solution in which
any composition can exist as a single homogeneous
phase. But at lower temperatures things get more
complicated.
Beneath a boundary called the
solvus
a 'miscibility
gap' appears. At these temperatures (for example
600 °C) the albite crystal structure is less tolerant of the
KAlSi
3
O
8
component (partly because of the large size
of the potassium atom), and at a KAlSi
3
O
8
content of
about 20% (
f
2
= 80% NaAlSi
3
O
8
) becomes
saturated
with it. Any KAlSi
3
O
8
present beyond this limit is
forced to exist as a separate KAlSi
3
O
8
-rich feldspar
phase, whose composition can be found by extending
a tie-line to the left-hand limb of the solvus, cutting it
at
f
1
(about 65% KAlSi
3
O
8
or 35% NaAlSi
3
O
8
). This
potassium feldspar is itself saturated with NaAlSi
3
O
8
.
A homogeneous alkali-feldspar solid solution such
as
f
h
ceases to be stable as it cools through the solvus. At
point
f
, for example, it is well inside the two-phase
region. Such a point represents, at equilibrium, a mix-
ture of
two
phases. The initially homogeneous feldspar
therefore breaks down, or
exsolves
, into two separate
phases
f
1
and
f
2
. But solid-state diffusion is too slow to
allow a cooling feldspar crystal to sort itself out into
two separate crystals. The usual result of exsolution is
a series of thin, lamellar domains of one phase enclosed
within a host crystal of the other. The lever rule (Box 2.3)
tells us that in the present example
f
1
will be more
abundant than
f
2
, and the cooling of crystal
f
h
will there-
fore produce a host crystal of composition
f
l
containing
exsolution lamellae
of phase
f
2
. Such structures are char-
acteristic of alkali feldspars, where they are known as
perthites
. Figure 2.7 shows a crystal of perthite viewed
in a polarizing microscope configured to highlight this
texture. The dark streaks are albite lamellae; the lighter
host is orthoclase (divided into upper and lower por-
tions that differ in shade owing to the
twinning
of the
crystal). Exsolution textures analogous to perthite
(although not given this name) are developed in some
pyroxenes (Plate 3), owing to a similar miscibility gap
between diopside (CaMgSi
2
O
6
) and enstatite (Mg
2
Si
2
O
6
).








































































































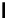





















































Search WWH ::

Custom Search