Image Processing Reference
In-Depth Information
These two equations are usually not verified by strictly monotonic, archimedean
t-norms and t-conorms.
Any strictly monotonic, archimedean t-norm (or t-conorm) can be defined based
on multiplicative generators, by equivalence with additive generators [CHE 89]:
[0
,
1]
2
,t
(
x, y
)=
h
−
1
h
(
x
)
h
(
y
)
,
∀
∈
(
x, y
)
[8.61]
where
h
is a strictly increasing function of [0
,
1] into [0
,
1] such that
h
(0) = 0 and
h
(1) = 1. The equivalence with the additive form is obtained simply by defining:
h
=
e
−
f
[8.62]
where
f
is an additive generating function.
The most common t-norms and t-conorms in this class are the product and the
algebraic sum:
[0
,
1]
2
,t
(
x, y
)=
xy,
∀
(
x, y
)
∈
T
(
x, y
)=
x
+
y
−
xy.
[8.63]
The only rational t-norms of this class are the Hamacher t-norms defined by
[HAM 78]:
xy
[0
,
1]
2
,
∀
(
x, y
)
∈
xy
)
,
[8.64]
γ
+(1
−
γ
)(
x
+
y
−
where
γ
is a positive parameter (for
γ
=1we get the product again). They are illus-
trated in Figure 8.5.
Figure 8.5.
Two examples of Hamacher t-norms, for
γ
=0
(left)
and
γ
=0
.
4
(right)











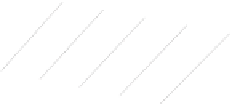







































































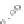
































































































































Search WWH ::

Custom Search