Beams - Mechanics of Structures: Variational and Computational Methods - page 749

engineering beam theory, including shear deformation coefficients. These shear coefficients,
α k s form a symmetric tensor. In general, the

principal axes of this tensor differ from the principal axes of the tensor for the moments
of inertia that appear in the beam bending equations. That is, the principal shear axes
differ from the principal bending axes, with symmetric cross-sections being an exception.
These shear deformation coefficients differ from the more traditional ones in that they vary
with the thickness of a cross section. Whereas a serious computational problem called shear
locking (see Chapter 13) can occur when the traditional shear deformation coefficients are
employed, the use of thickness-dependent coefficients tends to counter the problem.

ij ,i, j

= y, z , where, for example, α = 1 /

z, z

EXAMPLE 12.1 Finite Element Solution for Cross-Sectional Properties of a Symmetric Channel

Section
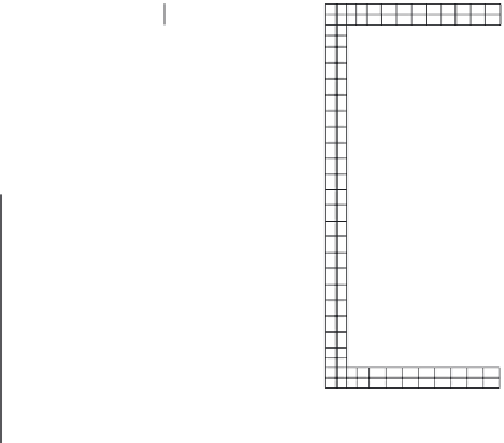
A symmetric open-channel section is shown in Fig. 12.12a. Let h

= 18 in., b = 8in . ,t =

1 in., and The reference coordinate system is at the midpoint of the web. The cross-

sectional properties and stresses can be calculated using a finite element program that can
treat a cross section of any shape (Pilkey, 2002).
The mesh chosen for calculations is shown in Fig. 12.12b. Some of the cross-sectional
properties computed using the finite element program are shown in Fig. 12.13.
Many references provide formulas to approximate several of the cross-sectional proper-
ties. Typically the torsional constant J for open thin-walled cross sections is usually given
by the approximate formula

ν = 1 / 3 .

St 3
3

J = (1)

where t is constant and S is the length of the median line of the cross section. For this
example, S 33 in 4 . The finite element calculation gives J 29 in 4 . = 34 in. Then, J = 11 . = 11 .

h

y

t

b

(b) Mesh

z

(a)

FIGURE 12.12
Channel section.