Information Technology Reference
In-Depth Information
Definition 6
([
23
]) A U-reduct is a minimal condition attribute subset
A
ↆ
C
preserving the following condition:
UA
A
(
X
i
)
=
UA
C
(
X
i
)
for all
i
∈
V
d
.
(U)
By definition, the classification ability of the upper approximations is equal to
that of the generalized decision function. Hence, condition (
U
) is equivalent to (
G
),
and we have that a U-reduct is a G-reduct and vice versa.
From Eqs. (
7.2
) and (
7.5
), we know that lower approximations are obtained from
upper approximations as well as from boundaries. This fact implies that each of the
preservation of all upper approximations or the preservation of all boundaries entails
the preservation of all lower approximations.
To sum up the above discussion, we have the next theorem.
Theorem 1
([
23
,
45
])
Let A be a subset of C . We have the following statements.
(a)
A is a Q-reduct if and only if A is an L-reduct.
(b)
A is a P-reduct if and only if A is an L-reduct.
(c)
A is a G-reduct if and only if A is a U-reduct.
(d)
A is a B-reduct if and only if A is a U-reduct.
(e)
A is a U-reduct as well as B-reduct, then A satisfies condition
(
L
)
.
All statements in the theorem can be easily proved by the equations which
appeared in Sect.
7.2.2
. For example, to prove Theorem
1
(d), we show that pre-
serving all boundaries implies preserving all upper approximations by Eq. (
7.3
), and
show the converse by Eq. (
7.6
).
From Theorem
1
(e), if
A
is a U-reduct then there exists an L-reduct
B
A
.Note
that the converse is not always true, i.e., for an L-reduct
B
, there is no guarantee that
there exists a U-reduct
A
ↆ
B
.
The relations of 6 types of reducts are depicted in Fig.
7.1
. Reducts located in
the upper part of the figure preserve regions much more. Therefore, such reducts are
larger in the sense of the set inclusion than the other reducts located in the lower part.
A line segment connecting two types of reducts implies that, for each reduct of the
upper type say
A
satisfies the preserving condition of the reduct of the lower one.
From the figure, we know that there are 2 different types of reducts: U-reducts and
L-reducts, and U-reducts are stronger than L-reducts.
ↇ
Remark 3
As shown in Theorem
1
, the six types of reducts are reduced to two types.
However, it is important to define all possible types of reducts and organize them
because of two reasons. One is that when we should mention different definitions of
Fig. 7.1
Strong-weak
hierarchy of 6 types of
reducts in RSM
strong
weak







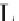





Search WWH ::

Custom Search