Polynomial chaos expansions and stochastic finite-element methods - Risk and Reliability in Geotechnical Engineering - page 290

# 1 # 2 # 3

y

y

y

x x x

# 4 # 5 # 6

y

y y

x x x

# 7 # 8 # 9

y

y y

x

x

x

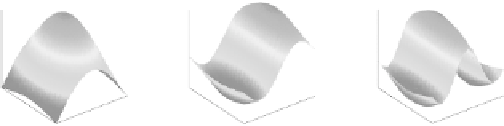
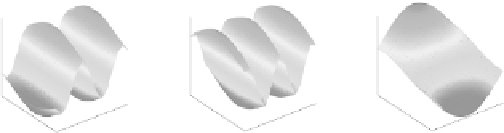
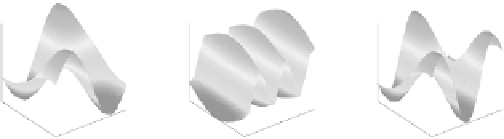
Figure 6.9 Example #3: First modes of the KL expansion of Young's modulus. (After Blatman, G. and B.
Sudret. 2011a. J. Comput. Phys. 230, 2345-2367.)

of the spatially variable random Young's modulus. Note that some modes have a zero total
Sobol' index, namely modes #2, 4, 7, and 8. From Figure 6.10 , it appears that they corre-
spond to antisymmetric modes with respect to the vertical axis (see Figure 6.9 ) . This means
that the symmetry of the problem is accounted for in the analysis. It is now clear why the
PC expansion is sparse since roughly half of the modes (i.e., half of the input variables of the
uncertainty quantification problem) do not play any role in the analysis.

6.5.4 Conclusions
In this section, three different problems of interest in geotechnical engineering have been
addressed, namely, the bearing capacity of a strip footing, the maximal settlement of a foun-
dation on a two-layer soil mass, and the settlement in case of a single layer with spatially
varying Young's modulus. In the first two cases, reliability analysis is carried out as a post-
processing of a PC expansion. The results in terms of probability of failure compare very
well with those obtained by reference methods such as IS at the design point. From a broader
experience in structural reliability analysis, it appears that PC expansions are suitable for
reliability analysis as long as the probability of failure to be computed is larger than 10 −5 .
For very small probabilities, suitable methods such as adaptive Kriging would rather be used
(Dubourg et al., 2011; Sudret, 2012).
In the last example, the spatial variability of the soil properties is introduced. The purpose
is to show that problems involving a large number of random input variables (here, 38) may
be solved at an affordable cost using sparse PC expansions (here, 200 samples in the ED).
Recent applications of this approach to the bearing capacity of 2D and three-dimensional
(3D) foundations can be found in Al Bittar and Soubra (2012); Mao et al. (2012).